On Mechanical Aids to Calculation
Edward Sang gave the lecture On Mechanical Aids to Calculation to the Actuarial Society of Edinburgh. His lecture was published in E Sang, 'On Mechanical Aids to Calculation. A Lecture to the Actuarial Society of Edinburgh', Journal of the Institute of Actuaries and Assurance Magazine 16 (4) (1871), 253-265. we give a version below.
On Mechanical Aids to Calculation. A Lecture to the Actuarial Society of Edinburgh.
By Edward Sang, F.R.S.E., Fellow of the Faculty of Actuaries in Scotland
In every branch of business, even in the very rudest stage of barter, men have to count. A skin is exchanged for so many cowries, a rifle for so many skins. Do what we will, go where we will, the necessity for counting meets us. Yet, though numbers be essential to all our operations, we do not easily form an idea of large numbers. Thus in no language do the separate names for numbers reach to twenty. In one, I believe, the numeration goes as far as fifteen; in the northern languages of Europe it reaches to twelve, but in most languages it stops short at ten.
More advanced numbers are held as composed of so many tens and so many units; they are named accordingly, as in English thirty-seven, meaning three tens and seven. In the Greek, Latin, and modern European languages, the names of the multiples of ten are derived from the names of the units; in some Asiatic languages the first five of them have independent names.
When we arrive at ten tens a new name, as hundred in English, is introduced, and we reckon in hundreds up to ten hundreds, which also receives a special name, with us thousand; and here, with only one exception that I know of, the special nomenclature ceases; all larger numbers being named by compounding the names of inferior numbers. In all this we see the use of helps to counting. Were numbers to receive independent names, the stock of words would be exhausted, the memory would fail to keep them in order, mistakes would be frequent. But the contrivance of articulate numeration introduces ease, clearness, and certainty.
As in the naming, so in the marking of numbers recourse was had to various contrivances for expediting the operations. The simple but prolix plan of putting down a counter for each article in barter, was soon improved by using a second kind of counter for each ten, or dozen, or score as the case might be. Thus in the very rudest kind of numeral notation which yet subsists, that used by the Romans, a stroke was made for each unit, and, to break the monotony, a cross stroke was used at the fifth, thus gradually giving rise to the use of the mark V for five and X for two fives or ten. The Arabs, however, adopted a more ingenious plan by using the first ten letters of their alphabet for the ten units; the second ten for the tens, and the third set of ten for the hundreds. A contrivance adopted by the Greeks, who, to supplement their defective alphabet, introduced some arbitrary marks. In India, however, the great improvement was made of using nine arbitrary marks for the nine units, and of indicating the tens, hundreds, and thousands by a change of position. This Hindi Rakkam was adopted by the Arabian arithmeticians, and by them introduced into Southern Europe; for which reason they are commonly but erroneously called by us Arabic numerals.
This Hindi contrivance, in daily use by all of us, is in reality a geometrical aid to counting: it takes a mechanical form in the shape of the swan-pan, or of the wire frame with strung beads.
When we mark down the successive numbers, the continual recurrence of the digits, 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 0, 1, 2, introduces the idea of circulation; and so leads us to divide the circumference of a wheel into ten parts and to write the numerals in them. In this way, by turning the wheel one step at a time and always in one direction we have the successive units. And here we have the first element in machine-counting.
By arranging a second wheel for the tens, and so placing it as that at each turn of the unit's wheel it shall be moved one step, we are enabled to count to one hundred; and by continuing this arrangement we can carry the numeration to any desired extent. Such is the construction of ordinary counting-machinery. In some of these machines the movements are continuously connected by means of toothed wheels, as in the cases of clock-work and of the ordinary gas-meter index. In others the motions are by jerks; the ten's wheel remains at rest until the units are passing from 9 to 0, at which time the tens are advanced one step; and the indication changes from say 69 to 70. Some of these latter machines are so contrived as that the wheels are locked until the carrying take place. Such are the machines used for registering the number of operations performed by the bank-note printing press. From their very construction continuously connected machines are always locked.
Such counting machines are of great use in many situations, as at loading and landing wharves, in large warehouses, at turnpike gates and in general wherever extensive tale has to be made, or wherever a check is required upon operations. They receive various forms according to the purpose for which they are intended; and by modifications and extensions they become the calculating machines to which our attention is principally to be directed.
As soon as we attempt to use a counting machine we find that there must be some provision for setting it to zero, or to whatever may be the number from which we wish to begin. This provision is made in two ways. In one the index is fitted stiffly, not permanently, to its axis, so that it may be turned round whenever a sufficient pressure is applied to it. We have a familiar example of this in our clocks and watches: without it we should not be able to correct the error of the time-keeper. In the other way the circumference of the index wheel is notched and a knuckled holder enters into the notch, being kept there by a spring. By this arrangement the wheel cannot be turned either backwards or forwards without raising the holder, so that it is kept steadily in its place until a pressure be applied sufficient to overcome the elasticity of the spring. Moreover, as soon as the tooth of the wheel is brought past the angle of the knuckle, the index is thrown forward or backward to the next number. In such a case the index wheels are independent of each other; but complete independence would not subserve our purpose; wherefore a provision is made to cause an advance of unit on any one index when the index below passes from 9 to 0.
An instrument arranged in this way may be set to any initial number and may be used at once for counting. But it is now capable of much more extensive use.
If I turn the unit's index forward seven steps I shall add seven to the indication, for should the units overpass the 9, as say from 8 to 5, the ten's index would be brought forward one step by means of the carrier. If I now turn the ten's index four steps forward, I shall add forty to the indication, that is to say, I shall have added forty-seven to the previously recorded number. In this way the instrument has become an addition machine; its action contains all that is essential to this operation. It is also a subtraction-instrument; the very movements which perform addition may be made to perform subtraction, and even at the same time. All that is needed is to inscribe on the indices numbers in the inverse order.
Various contrivances have been made for the purpose of facilitating the addition; in all of these the object aimed at is to have the addend set upon the machine, and the summation performed by the motion of the instrument, generally by one turn of the handle. Much ingenuity has been expended on these contrivances, and considerable apparent diversity of arrangement has been the result; yet the essential characters of the action are the same in all.
We desire, for example, to bring the ten's index forward four steps by one action. For this purpose we may arrange that a hook or propeller shall have a stroke of four divisions; or we may cause four teeth of some wheel to act upon the index: both of these methods are in use; the latter is seen in Thomas' machine; the former in the striking part of our common house clocks, to which I shall have occasion afterwards to revert.
In Thomas' machine there is placed a cylinder to make a complete turn at each operation; on its surface there are fixed nine parallel bars, or as we may call them elongated teeth, which are to work the teeth of the counting wheel: the lengths of these bars are equi-different, so that at one place of the cylinder the whole nine are ready to act, at the next place eight, and so on. The counting wheel is made to slip longitudinally upon a squared axis, so that we can bring it opposite to any desired part of the cylinder.
If we bring the counting wheel so as to be acted on by four bars, one turn of the cylinder will cause an addition of four, and each succeeding turn will cause a new addition of four.
Now for each of the indices, that is for units, tens, hundreds, and so on, we have a special cylinder, and therefore if we set the counting wheels to specified digits, and turn each of the cylinders once round (for the present we shall suppose once in succession) we shall have added the specified number to the previous indication.
If we do this for one cylinder after another some considerable time will be required. The contrivance for economising that time is exceedingly ingenious in Thomas' machine. He causes all the cylinders to be actuated at once by the working handle, so that all the additions go on nearly at once. This, however, would cause confusion in the carrying. He therefore makes the index wheels entirely independent of each other, and arranges the carrying in another way. On each index wheel there is fixed a stud to come in contact with a lever whenever the indication passes from 9 to 0, and so to push this lever aside; and a detent is provided to keep this lever back after the stud has passed onwards. This lever brings into action the carrier fixed on the axis, and this carrier only acts after the addition by the bars has been completed. Thus by arranging the cylinders one tooth in arrear at each step, the carrying from one rank to another is completely effected, and the whole addition completed by one turn of the handle.
By help of this machine, then, we can perform addition, and by turning the handle repeatedly, we can perform successive additions, and so form an equi-different progression.
On the same instrument there is an arrangement, by means of bevelled wheels, for changing the direction of the motion of the indices, and thereby converting addition into subtraction.
M Thomas has provided the means for shifting the addend or subtrahend to a different position on the numeration scale. This he accomplishes by sliding the whole frame carrying the indices sideways, at the same time preserving the recorded number. By this means, when we have set the addend to say 3734, we are able, by shifting the index frame two steps to the right, to convert the addend into 373400. He has also arranged a set of indicators to show how many times the addition or subtraction has been performed in the various positions, and so to show the multiplier.
This very ingeniously-constructed instrument exhibits the various characters and requirements of a calculating machine, in a tolerably complete state of development. After all, however, it is only an addition-machine, the only multiplication which it performs being that by the powers of ten, done by the transposition the frame. A multiplying-machine proper would be such, that when the two factors are set upon the instrument, their product should be exhibited automatically. As yet, we have scarcely any approach to such an instrument.
The first attempt at an apparatus for multiplying was made by John Napier, the inventor of logarithms; and the study of this attempt may serve to throw considerable light upon the general subject of mechanical aids to calculation. The contrivance is known under the name of Napier's Bones, and is described in a work called Rhabdologia. It is simply a moveable multiplication table. The multiplicand is written on the top of a rod or other moveable slip, its multiples in lines below, the units being separated from the tens by diagonal lines; and these slips are sufficiently numerous to allow of the formation of any number by their initial figures. If the multiplicand be, for example, 43429448, we arrange slips showing that number at the top, and then in any of the horizontal lines we have its multiple by carrying the tens on one slip to the units of that on its right. In this way the computer obtains the successive lines of his product: these he has to write down and add together in the usual way. I am not acquainted with any other contrivance for showing the product of two numerical factors. There exist, however, several instruments for showing the product of factors represented geometrically, and for the summation of such products. It may be useful to examine these, in order to understand the kind of instrument that is desirable for purely arithmetical operations.
In order to obtain the surface of an irregular figure drawn upon paper, we divide it into a number of parts by parallel lines, and get the area of each of these by multiplying the length by the breadth; and finally we add all these products together. The instruments to which I allude perform all of these operations, and show the result at once. In order to understand how this is accomplished, we shall draw a straight line outside of the figure, and bring the parallels to cut it, as in the example. Here we at once perceive that the surface of the figure is the sum of all the rhomboids, such as , drawn to the farther side of the contour, less the sum of all such as drawn to its inner side.
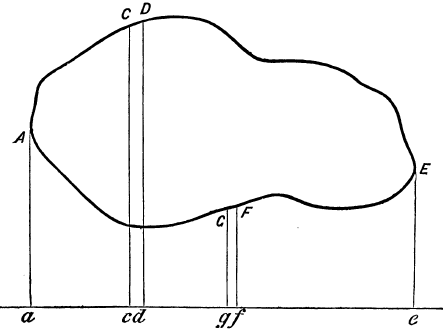
In order to represent mechanically the areas of such rectangles we must have a motion whose extent is in the compound ratio of the breadth , and of the length . In what is, I believe, the first machine of this kind ever contrived, the Platometer of Mr John Sang, this compounding of two ratios is accomplished by help of a cone, mounted on two wheels. Let us suppose that the axis of the cone is laid in the direction , and that, in rolling, its apex describes the line , then while it moves from to , the quantity of turning is proportional to . But the surface of the cone opposite C D must move through a distance also proportional to , wherefore, the actual motion there must be proportional to the surface of the rectangle . This motion is measured and recorded by a wheel rolling on the cone, and capable of being slid upon it lengthways. Thus when the tracer of the instrument is led along the contour , the wheel measures and adds up all the minute rectangles from to . Similarly when the tracer is led along the other part of the boundary from to , the same wheel measures all the rectangles , but now in the opposite or subtractive direction; so that when the tracer is brought back to , the index shows the number of square inches in the surface of the figure.
The Platometer contrived by Mr Beverley, of Dunedin, New Zealand, exhibits the same principle brought out in a different way. If a cylinder be dragged along a surface endwise it does not turn; if it be moved in a direction at right angles to its axis it rolls; in other positions it partly slides and partly rolls, and in general the quantity of turning is proportional to the sine of the angle between the direction of the axis and the direction of the motion. Taking advantage of this law Mr. Beverley arranges his instrument so that the quantity of angular motion is proportional to the distance of the tracer from a certain straight line; it is, at the same time, proportional to the extent of the actual displacement, and hence the indication is proportional to the area of the rectangle.
These instruments are called Platometers because of the use to which they have been applied; they are in reality integrating machines, and may be applied to a great variety of purposes.
Thus, let us take Mr Sang's Platometer, and instead of making it to roll like a carriage on wheels, let us fix the frame, leaving the wheels and cone free to turn; and seek to apply it to the solution of such a problem as this, "to find the value of an assurance." We shall divide along the periphery of one of the wheels parts to represent £1; and in the direction of the slope of the cone, other parts to represent the decreasing values of £l payable years hence. The product of £1 payable for each person who dies in the year, into the value of £1 for that year is obtained by bringing the index wheel to the proper distance from the apex of the cone, and then turning the cone round by as many divisions as there are deaths in the mortality table. And if we perform this operation regularly for each year during the whole of the table, the index wheel will at once record the sum total of all these products. Beverley's machine may easily be arranged to do the same work.
Both of these instruments, however, and indeed all machines of this kind, depend on a combination of sliding and rolling, and are thus liable to considerable inexactitude in their indications. They fall far short of the certainty which attends the use of toothed wheels, and would be unfit for such calculations as come under the notice of the actuary. Having thus given a sketch of the general construction and mode of action of calculating machines, I proceed to consider how these instruments are to be applied and what is the amount of help to be expected from them.
Beginning with the earliest contrivance, that by Napier, let us place a set of his rods before us, and proceed to make use of them in calculation. We wish, for example, to multiply 397364 by 7. Having collected and arranged the sticks headed 3, 9, 7, 3, 6, 4, we pass down to the seventh row and there find
| 2 / 1 | 6 / 3 | 4 / 9 | 2 / 1 | 4 / 2 | 2 / 8 |
giving the result 2781548. Now the very least attention is enough to show that the trouble of seeking out the sticks, of arranging them, and of writing down the product, must be many times greater than that of writing down the product without the sticks. It must, not, however, be thought that Napier's rods were of no use: in his day men learned to count after they were grown old, the Indian numerals were novelties, and the multiplication table was by no means at the finger-ends of computers; thus the rods were then of real use even for a solitary operation such as this.
When we have to find the product of two numbers, each of several digits, the utility of the contrivance is better seen. Thus, if the product of the above number by 782935 were wanted, one arrangement of the rod serves for all the six multipliers, and the six products may be easily written down for addition. There can be no doubt but that this was a considerable aid to those who were unaccustomed to the use of numbers, and on whom the necessity for heavy work had come like an oppression. Astronomy was beginning to assume an exact form, trigonometrical tables had been extended to seven decimal places; the dimensions of the planetary orbits had been ascertained, and even their eccentricities with considerable precision. Hence just as the need for trigonometrical calculations grew greater, the number of decimal places also increased, and the burden of the multiplications and divisions became so intolerable that even Kepler exclaimed, "The duration of human life is too short to meet the requirements of the astronomer." Under such circumstances, the assistance to be obtained from Napier's rods was by no means to be despised.
Now-a-days, however, an ordinarily good computer, and particularly one who has acquired the easy art of working from the left hand, finds that the time occupied by the mental operation is but an insignificant fraction of that taken up by the manual operation of writing; and to him a set of Napier's rods would only prove a troublesome incumbrance.
Thus we can only estimate the value of any such mechanical aid, by taking into account the attainments of the operator. To those who can hardly tell whether seven times nine make sixty-five or sixty-three, a set of Napier's rods may safely be recommended; yet even to such a one the evil may overbalance the good, since he will be prevented from acquiring expertness through practice.
Passing from the elementary contrivance of Napier to the elaborate and ingeniously contrived machine of Thomas, we have to arrange the indices so as to show the multiplicand, setting at the same time the result indices to zero. On now turning the handle one, two, three times we obtain the product of the multiplicand by the successive digits one, two, three. Having thus obtained the product by the units, we shift the apparatus one step along, turn the handle once for every figure in the tens, so on for the hundreds, until the multiplication be completed: the result has now to be inspected and written down upon our paper. In order to prevent mistake, the multiplier actually used is recorded by the machine, this record has also to be inspected and compared with our data.
The question whether or not this machine manipulation be a help to the computer has to be settled by two considerations; one, the ease of managing the machine, the other, the attainments of the computer himself; it is therefore not susceptible of a general answer.
The transference of the factors from the paper to the machine, the comparisons needed to prevent mistake, and the transference of the result from the machine are serious inconveniences, which appear the greater when we consider that a computer is far more liable to make a mistake in copying than to commit an error in calculation. When we take into account the many short cuts and the abbreviations occurring in general practice, and consider that these are quite lost in the machine work, we begin to think that the balance may not be quite so much in favour of the machine as appears at the first sight of it; and may even be inclined to enquire whether, to an expert computer, the instrument would not prove to be a serious hindrance. And then there is one obvious drawback, that the use of such an aid will not tend to make us good computers.
The application of this machine to division, consists merely in successive subtractions. You by one turn of the handle subtract the divisor, shifted to the proper step on the numeration scale, once from the dividend, and you continue this until the remainder be less than the subtrahend. Having thus discovered the highest digit of the quotient, you shift the dividend one step lower - repeat the subtractions and so continue till the last figure of the quotient appears.
This process contrasts much less favourably than that for multiplying with the ordinary paper work: indeed, to a moderately rapid computer it is irksome, and for this reason that attention has to be given to each successive remainder, lest we should turn the handle once too often; we have, in fact, much more than the mental fatigue of a common division.
The advantages of calculating machines in multiplying or dividing may with some be a matter of doubt; but there can only be one opinion when we apply them to desultory additions or subtractions. We have to add one number to another, or we have to sum up a column of numbers. Having brought the result indices to zero, we set the first addend upon the machine and turn the handle; then set the second addend and give another turn; and so on to the end of the chapter. Why, the time spent in setting the indices would greatly exceed that needed for running up the column and inscribing the result.
The great benefit to be conferred upon us by calculating machines has always been looked for in the compilation of tables; and that benefit is expected in two ways, one in rapidity, and one in certainty of operation.
The simplest kind of table is that of equi-different quantities, such as multiples of the modulus of denary logarithms, the lengths of circular arcs; and for this work the machine seems to be admirably contrived. We have only to put the indices to the common difference, and then each turn of the handle will produce a new term in the series; with a rapidity with which the hand cannot compute, and also with, if the machine be properly made, absolute certainty. True; but where is this result? It is shown on the moveable indices, and, to be of any use to us, must be copied upon paper. Now, the time expended in this copying will be considerably greater than that needed for summing and writing the result in the ordinary way: and although the indication be correct, the copy of it is liable to error; while, if the addition be made continuously on paper, and tested in the usual way, we are almost absolutely freed from error, because an error would necessarily be continued, and so could not escape notice.
In order to obtain the advantage of the certainty of machine action, the machine itself must make its marks upon the paper. Such is the action of numbering machines for railway tickets, for pages of books, and the like. A proper calculating machine for this class of tabular work, punching the results upon a plate, or operating by help of a type-composing machine, would undoubtedly produce trustworthy results.
Nor is the table of equi-different quantities the only one to which machinery may be applied. In our house clocks we have an arrangement whereby changing differences are obtained; these differences increasing by unit each hour. So, by causing the indices of the addend to change at each step, that is, by introducing second differences, we may construct tables of a higher order, such as tables of squares. And again, by employing a third addition table to change the second difference, we may construct tables of cubes. And thus, in general, calculating machines may be made to compute and tabulate the values of all algebraic functions having integer indices. But even such machines are only advantageous when they entirely dispense with pen work.
The great mass of tables, costing labour and thought to the computer, such as trigonometrical canons, tables of logarithms, have their orders of differences interminate, and so it is impossible to compute them by machinery. For then the differences of the last available order must be computed intellectually, and then, indeed, we might set up the type by machinery, provided each successive difference were set by hand. The instrument would therefore fall to be classed among composing machines.
Thus, on the whole, arithmeticians have not much to expect from the aid of calculating machines. A few tables, otherwise very easily made, comprise the whole extent of our expected benefits; and we must fall back upon the wholesome truth that we cannot delegate our intellectual functions, and say to a machine, to a formula, to a rule, or to a dogma, I am too lazy to think, do please think for me.
Last Updated January 2021