Mathematics of the Incas
It is often thought that mathematics can only develop after a civilisation has developed some form of writing. Although not easy for us to understand today, many civilisations reached highly advanced states without ever developing written records. Now of course it is difficult for us to know much about such civilisations since there is no written record to be studied today. This article looks at the mathematical achievements of one such civilisation.
The civilisation we discuss, which does not appear to have found a need to develop writing, is that of the Incas. The Inca empire which existed in 1532, before the Spanish conquest, was vast. It spread over an area which stretched from what is now the northern border of Ecuador to Mendoza in west-central Argentina and to the Maule River in central Chile. The Inca people numbered around 12 million but they were from many different ethnic groups and spoke about 20 different languages. The civilisation had reached a high level of sophistication with a remarkable system of roads, agriculture, textile design, and administration. Of course even if writing is not required to achieve this level, counting and recording of numerical information is necessary. The Incas had developed a method of recording numerical information which did not require writing. It involved knots in strings called quipu.
The quipu was not a calculator, rather it was a storage device. Remember that the Incas had no written records and so the quipu played a major role in the administration of the Inca empire since it allowed numerical information to be kept. Let us first describe the basic quipu, with its positional number system, and then look at the ways that it was used in Inca society.
The quipu consists of strings which were knotted to represent numbers. A number was represented by knots in the string, using a positional base 10 representation. If the number 586 was to be recorded on the string then six touching knots were placed near the free end of the string, a space was left, then eight touching knots for the 10s, another space, and finally 5 touching knots for the 100s.
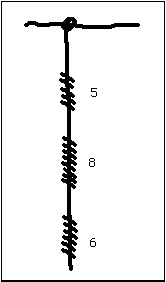
For larger numbers more knot groups were used, one for each power of 10, in the same way as the digits of the number system we use here are occur in different positions to indicate the number of the corresponding power of 10 in that position.
Now it is not quite true that the same knots were used irrespective of the position as would be the case in a true positional system. There seems only one exception, namely the unit position, where different styles of knots were used from those in the other positions. In fact two different styles were used in the units position, one style if the unit were a 1 and a second style if the unit were greater than one. Both these styles differed from the standard knot used for all other positions. The system had a zero position, for this would be represented as no knots in that position. This meant that the spacing had to be highly regular so that zero positions would be clear.
There are many drawings and descriptions of quipus made by the Spanish invaders. Garcilaso de la Vega, whose mother was an Inca and whose father was Spanish, wrote (see for example [5]):-
As well as the colour coding, another way of distinguishing the strings was to make some strings subsidiary ones, tied to the middle of a main string rather than being tied to the main horizontal cord.

We quote Garcilaso de la Vega again [5]:-
Much information on the quipus comes from a letter of the Peruvian Felipe Guaman Poma de Ayala to the King of Spain, written about eighty years after the Spanish conquest of the Incas. This remarkable letter contains 1179 pages and there are several drawings which show quipus. A fascinating aspect of one of these drawing is a picture of a counting board in the bottom left hand corner of one of them. This is called the yupana and is presumed to be the counting board of the Incas.
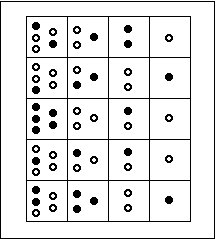
Interpretations of how this counting board, or Peruvian abacus, might have been used have been given by several authors, see for example [9] and [11]. However some historians are less certain that this really is a Peruvian abacus. For example [2] in which the authors write:-
The civilisation we discuss, which does not appear to have found a need to develop writing, is that of the Incas. The Inca empire which existed in 1532, before the Spanish conquest, was vast. It spread over an area which stretched from what is now the northern border of Ecuador to Mendoza in west-central Argentina and to the Maule River in central Chile. The Inca people numbered around 12 million but they were from many different ethnic groups and spoke about 20 different languages. The civilisation had reached a high level of sophistication with a remarkable system of roads, agriculture, textile design, and administration. Of course even if writing is not required to achieve this level, counting and recording of numerical information is necessary. The Incas had developed a method of recording numerical information which did not require writing. It involved knots in strings called quipu.
The quipu was not a calculator, rather it was a storage device. Remember that the Incas had no written records and so the quipu played a major role in the administration of the Inca empire since it allowed numerical information to be kept. Let us first describe the basic quipu, with its positional number system, and then look at the ways that it was used in Inca society.
The quipu consists of strings which were knotted to represent numbers. A number was represented by knots in the string, using a positional base 10 representation. If the number 586 was to be recorded on the string then six touching knots were placed near the free end of the string, a space was left, then eight touching knots for the 10s, another space, and finally 5 touching knots for the 100s.

586 on a quipu.
For larger numbers more knot groups were used, one for each power of 10, in the same way as the digits of the number system we use here are occur in different positions to indicate the number of the corresponding power of 10 in that position.
Now it is not quite true that the same knots were used irrespective of the position as would be the case in a true positional system. There seems only one exception, namely the unit position, where different styles of knots were used from those in the other positions. In fact two different styles were used in the units position, one style if the unit were a 1 and a second style if the unit were greater than one. Both these styles differed from the standard knot used for all other positions. The system had a zero position, for this would be represented as no knots in that position. This meant that the spacing had to be highly regular so that zero positions would be clear.
There are many drawings and descriptions of quipus made by the Spanish invaders. Garcilaso de la Vega, whose mother was an Inca and whose father was Spanish, wrote (see for example [5]):-
According to their position, the knots signified units, tens, hundreds, thousands, ten thousands and, exceptionally, hundred thousands, and they are all well aligned on their different cords as the figures that an accountant sets down, column by column, in his ledger.Now of course recording a number on a string would, in itself, not be that useful. A quipu had many strings and there had to be some way that the string carrying the record of a particular number could be identified. The primary way this was done was by the use of colour. Numbers were recorded on strings of a particular colour to identify what that number was recording. For example numbers of cattle might be recorded on green strings while numbers of sheep might be recorded on white strings. The colours each had several meanings, some of which were abstract ideas, some concrete as in the cattle and sheep example. White strings had the abstract meaning of "peace" while red strings had the abstract meaning of "war".
As well as the colour coding, another way of distinguishing the strings was to make some strings subsidiary ones, tied to the middle of a main string rather than being tied to the main horizontal cord.

Quipu with subsidiary cords.
We quote Garcilaso de la Vega again [5]:-
The ordinary judges gave a monthly account of the sentences they imposed to their superiors, and they in turn reported to their immediate superiors, and so on finally to the Inca or those of his Supreme Council. The method of making these reports was by means of knots, made of various colours, where knots of such and such colours denote that such and such crimes had been punished. Smaller threads attached to thicker cords were of different colours to signify the precise nature of the punishment that had been inflicted.It was not only judges who sent quipus to be kept in a central record. The Inca king appointed quipucamayocs, or keepers of the knots, to each town. Larger towns might have had up to thirty quipucamayocs who were essentially government statisticians, keeping official census records of the population, records of the produce of the town, its animals and weapons. This and other information was sent annually to the capital Cuzco. There was even an official delivery service to take to quipus to Cuzco which consisted of relay runners who passed the quipus on to the next runner at specially constructed staging posts. The terrain was extremely difficult yet the Incas had constructed roads to make the passing of information by quipus surprisingly rapid.
Much information on the quipus comes from a letter of the Peruvian Felipe Guaman Poma de Ayala to the King of Spain, written about eighty years after the Spanish conquest of the Incas. This remarkable letter contains 1179 pages and there are several drawings which show quipus. A fascinating aspect of one of these drawing is a picture of a counting board in the bottom left hand corner of one of them. This is called the yupana and is presumed to be the counting board of the Incas.

This is what the yupana looked like.
Interpretations of how this counting board, or Peruvian abacus, might have been used have been given by several authors, see for example [9] and [11]. However some historians are less certain that this really is a Peruvian abacus. For example [2] in which the authors write:-
It is unclear from Poma's commentary whether it is his version of a device associated with Spanish activities analogous to those of the person depicted or whether he is implying its association with the Incas. In either case, his commentary makes interpretation of the configuration and the meaning of the unfilled and filled holes highly speculative.It is a difficult task to gain further insights into the mathematical understanding of the Incas. The book [6] by Urton is interesting for it examines the concept of number as understood by the Inca people. As one might expect, their concept of number was a very concrete one, unlike our concept of number which is a highly abstract one (although this is not really understood by many people). The concrete way of conceiving numbers is illustrated by different words used when describing properties of numbers. One example given in [6] is that of even and odd numbers. Now the ideas of an even number, say, relies on having an abstract concept of number which is independent of the objects being counted. However, the Peruvian languages had different words which applied to different types of objects. For example separate words occur for the idea of [6]:-
... the two together that make a pair ...This is a fascinating topic and one which deserves much further research. One wonders whether the Incas applied their number system to solve mathematical problems. Was it merely for recording? If the yupana really was an abacus then it must have been used to solve problems and this prompts the intriguing question of what these problems were. A tantalising glimpse may be contained in the writings of the Spanish priest José de Acosta who lived among the Incas from 1571 to 1586. He writes in his book Historia Natural Moral de las Indias Ⓣ which was published in Madrid in 1596:-
... the one together with its mate ...
... two - in reference to one thing that is divided into two parts ...
... a pair of two separate things bound intimately together, such as two bulls yoked together for ploughing ...
etc.
To see them use another kind of calculator, with maize kernels, is a perfect joy. In order to carry out a very difficult computation for which an able computer would require pen and paper, these Indians make use of their kernels. They place one here, three somewhere else and eight, I know not where. They move one kernel here and there and the fact is that they are able to complete their computation without making the smallest mistake. as a matter of fact, they are better at practical arithmetic than we are with pen and ink. Whether this is not ingenious and whether these people are wild animals let those judge who will! What I consider as certain is that in what they undertake to do they are superior to us.What a pity that de Acosta did not have the mathematical skills to give a precise description which would have allowed us to understand this method of calculation by the Incas.
References (show)
- M Ascher, Ethnomathematics : A multicultural view of mathematical ideas (Pacific Grove, CA, 1991).
- M Ascher and R Ascher, Code of the quipu : A study in media, mathematics, and culture (Ann Arbor, Mich., 1981).
- M Ascher and R Ascher, Mathematics of the Incas: code of the Quipu (Mineola, NY, 1997).
- G Ifrah, A universal history of numbers : From prehistory to the invention of the computer (London, 1998).
- G G Joseph, The crest of the peacock (London, 1991).
- G Urton, The social life of numbers : A Quechua ontology of numbers and philosophy of arithmetic (Austin, TX, 1997).
- M Ascher, Mathematical ideas of the Incas, in Native American mathematics (Austin, TX, 1986), 261-289.
- M Ascher, The logical-numerical system of Inca quipus, Ann. Hist. Comput. 5 (3) (1983), 268-278.
- W Burns Glynn, Calculation table of the Incas (Spanish), Bol. Lima No. 11 (1981), 1-15.
- A Christensen, The Peruvian quipu, in History and science of knots (River Edge, NJ, 1996), 71-88.
- D Pareja, Pre-Hispanic tools of computation : the quipu and the yupana (Spanish), Rev. Integr. Temas Mat. 4 (1) (1986), 37-56.
- W S Sizer, Mathematical notions in preliterate societies, Math. Intelligencer 13 (4) (1991), 53-60.
Written by J J O'Connor and E F Robertson
Last Update January 2001
Last Update January 2001