Curves
Freeth's Nephroid
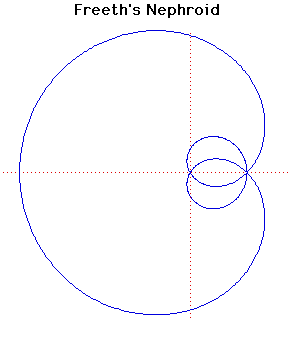
Description
This is a strophoid of a circle with the pole at the centre of the circle and the fixed point on the circumference of the circle.In the picture above, is the origin and is the node where the curve crosses itself three times.
If the line through parallel to the -axis cuts the nephroid at then angle is 3π /7 . This can be used to construct a regular 7 sided figure.
T J Freeth (1819-1904) was an English mathematician. In a paper published by the London Mathematical Society in 1879 he described various strophoids, including the strophoid of a trisectrix.