MacTutor logo MacTutor

Curves

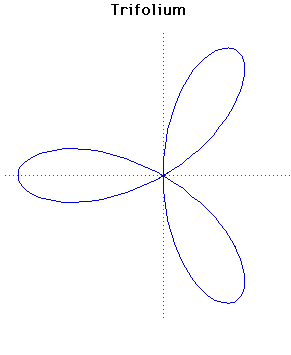
Trifolium

Main
Cartesian equation:
(x2+y2)(y2+x(x+a))=4axy2(x^{2} + y^{2})(y^{2} + x(x + a)) = 4axy^{2}
Polar equation:
r=acos⁡θ(4sin⁡2θ−1)r = a \cos \theta (4\sin^{2} \theta - 1)

View the interactive version of this curve.

Description

The general form of the folium is given by the formula
(x2+y2)(y2+x(x+b))=4axy2(x^{2} + y^{2})(y^{2} + x(x + b)) = 4axy^{2}
or, in polar coordinates
r=−bcos⁡θ+4acos⁡θsin⁡2θr = -b \cos\theta + 4a \cos\theta \sin^{2}\theta.
The word folium means 'leaf-shaped'.

There are three special forms of the folium, the simple folium, the double folium and the trifolium. These correspond to the cases
b=4a,b=0,b=ab = 4a, b = 0, b = a
respectively in the formula for the general form.

The graph plotted above is the trifolium. There are separate entries for the simple folium and the double folium.

Associated Curves

Definitions of the Associated curves

  • Evolute
  • Involute 1
  • Involute 2
  • Inverse curve wrt origin
  • Inverse wrt another circle
  • Pedal curve wrt origin
  • Pedal wrt another point
  • Negative pedal curve wrt origin
  • Negative pedal wrt another point
  • Caustic wrt horizontal rays
  • Caustic curve wrt another point