The brachistochrone problem
The brachistochrone problem was posed by Johann Bernoulli in Acta Eruditorum Ⓣ in June 1696. He introduced the problem as follows:-

Johann Bernoulli was not the first to consider the brachistochrone problem. Galileo in 1638 had studied the problem in his famous work Discourse on two new sciences. His version of the problem was first to find the straight line from a point to the point on a vertical line which it would reach the quickest. He correctly calculated that such a line from to the vertical line would be at an angle of 45 reaching the required vertical line at say.
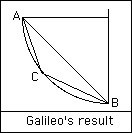
He calculated the time taken for the point to move from to in a straight line, then he showed that the point would reach more quickly if it travelled along the two line segments followed by where is a point on an arc of a circle.
Although Galileo was perfectly correct in this, he then made an error when he next argued that the path of quickest descent from to would be an arc of a circle - an incorrect deduction.
Returning to Johann Bernoulli he stated the problem in Acta Eruditorum Ⓣ and, although knowing how to solve it himself, he challenged others to solve it. Leibniz persuaded Johann Bernoulli to allow a longer time for solutions to be produced than the six months he had originally intended so that foreign mathematicians would also have a chance to solve the problem. Five solutions were obtained, Newton, Jacob Bernoulli, Leibniz and de L'Hôpital solving the problem in addition to Johann Bernoulli.
Now Johann Bernoulli and Leibniz deliberately tempted Newton with this problem. It is not surprising, given the dispute over the calculus, that Johann Bernoulli had included these words in his challenge:-
See more about the cycloid at THIS LINK.
The May 1697 publication of Acta Eruditorum Ⓣ contained Leibniz's solution to the brachistochrone problem on page 205, Johann Bernoulli's solution on pages 206 to 211, Jacob Bernoulli's solution on pages 211 to 214, and a Latin translation of Newton's solution on page 223. The solution by de L'Hôpital was not published until 1988 when, nearly 300 years later, Jeanne Peiffer presented it as Appendix 1 in [1]. Johann Bernoulli gave the solvers, saying:-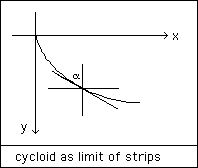
In the limit, as the strips become infinitely thin, the line segments tend to a curve where at each point the angle the line segment made with the vertical becomes the angle the tangent to the curve makes with the vertical. If is the velocity at and is the angle the tangent makes with the vertical then the curve satisfies
The cycloid satisfies this equation. To see this note that
Huygens had shown in 1659, prompted by Pascal's challenge about the cycloid, that the cycloid is the solution to the tautochrone problem, namely that of finding the curve for which the time taken by a particle sliding down the curve under uniform gravity to its lowest point is independent of its starting point.
Johann Bernoulli ended his solution of the brachistochrone problem with these words:-
The methods which the brothers developed to solve the challenge problems they were tossing at each other were put in a general setting by Euler in Methodus inveniendi lineas curvas maximi minimive proprietate gaudentes sive solutio problematis isoperimetrici latissimo sensu accepti Ⓣ published in 1744. In this work Euler generalises the problems studies by the Bernoulli brothers but retains the geometrical approach developed by Johann Bernoulli to solve them. He found what has now come to be known as the Euler-Lagrange differential equation for a function of the maximising or minimising function and its derivative.
The idea is to find a function which maximises or minimises a certain quantity where the function is constrained to satisfy certain constraints. For example Johann Bernoulli had posed certain geodesic problems to Euler which, like the brachistochrone problem, were of this type. Here the problem was to find curves of minimum length where the curves were constrained to lie on a given surface. Euler, however, commented that his geometrical approach to these problems was not ideal and it only gave necessary conditions that a solution has to satisfy. The question of the existence of a solution was not solved by Euler's contribution.
Lagrange, in 1760, published Essay on a new method of determining the maxima and minima of indefinite integral formulas. It gave an analytic method to attach calculus of variations type problems. In the introduction to the paper Lagrange gives the historical development of the ideas which we have described above but it seems appropriate to end this article by giving what is in effect a summary of the developments in Lagrange's words:-
I, Johann Bernoulli, address the most brilliant mathematicians in the world. Nothing is more attractive to intelligent people than an honest, challenging problem, whose possible solution will bestow fame and remain as a lasting monument. Following the example set by Pascal, Fermat, etc., I hope to gain the gratitude of the whole scientific community by placing before the finest mathematicians of our time a problem which will test their methods and the strength of their intellect. If someone communicates to me the solution of the proposed problem, I shall publicly declare him worthy of praise.The problem he posed was the following:-
Given two points A and B in a vertical plane, what is the curve traced out by a point acted on only by gravity, which starts at A and reaches B in the shortest time.Perhaps we are reading too much into Johann Bernoulli's references to Pascal and Fermat, but it interesting to note that Pascal's most famous challenge concerned the cycloid, which Johann Bernoulli knew at this stage to be the solution to the brachistochrone problem, and his method of solving the problem used ideas due to Fermat.

Johann Bernoulli was not the first to consider the brachistochrone problem. Galileo in 1638 had studied the problem in his famous work Discourse on two new sciences. His version of the problem was first to find the straight line from a point to the point on a vertical line which it would reach the quickest. He correctly calculated that such a line from to the vertical line would be at an angle of 45 reaching the required vertical line at say.

He calculated the time taken for the point to move from to in a straight line, then he showed that the point would reach more quickly if it travelled along the two line segments followed by where is a point on an arc of a circle.
Although Galileo was perfectly correct in this, he then made an error when he next argued that the path of quickest descent from to would be an arc of a circle - an incorrect deduction.
Returning to Johann Bernoulli he stated the problem in Acta Eruditorum Ⓣ and, although knowing how to solve it himself, he challenged others to solve it. Leibniz persuaded Johann Bernoulli to allow a longer time for solutions to be produced than the six months he had originally intended so that foreign mathematicians would also have a chance to solve the problem. Five solutions were obtained, Newton, Jacob Bernoulli, Leibniz and de L'Hôpital solving the problem in addition to Johann Bernoulli.
Now Johann Bernoulli and Leibniz deliberately tempted Newton with this problem. It is not surprising, given the dispute over the calculus, that Johann Bernoulli had included these words in his challenge:-
...there are fewer who are likely to solve our excellent problems, aye, fewer even among the very mathematicians who boast that [they]... have wonderfully extended its bounds by means of the golden theorems which (they thought) were known to no one, but which in fact had long previously been published by others.According to Newton's biographer Conduitt, he solved the problem in an evening after returning home from the Royal Mint. Newton:-
... in the midst of the hurry of the great recoinage, did not come home till four (in the afternoon) from the Tower very much tired, but did not sleep till he had solved it, which was by four in the morning.Newton sent his solution to Charles Montague, the Earl of Halifax, who was an innovative finance minister and the founder of the Bank of England. Montague was the principal patron and lifelong friend of Newton and, in addition, the 'common-law' husband of Newton's niece. He was President of the Royal Society during the years 1695 to 1698 so it was natural that Newton send him his solution to the brachistochrone problem. However, as he wrote afterwards, the episode did not please Newton:-
I do not love to be dunned [pestered] and teased by foreigners about mathematical things ...The Royal Society published Newton's solution anonymously in the Philosophical Transactions of the Royal Society in January 1697. His solution was explained to Montague as follows:-

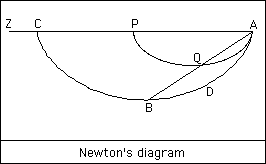
Problem. It is required to find the curve ADB in which a weight, by the force of its gravity, shall descend most swiftly from any given point A to any given point B.
Solution. From the given point A let there be drawn an unlimited straight line APCZ parallel to the horizontal, and on it let there be described an arbitrary cycloid AQP meeting the straight line AB (assumed drawn and produced if necessary) in the point Q, and further a second cycloid ADC whose base and height are to the base and height of the former as AB is to AQ respectively. This last cycloid will pass through the point B, and it will be that curve along which a weight, by the force of its gravity, shall descend most swiftly from the point A to the point B.
See more about the cycloid at THIS LINK.
The May 1697 publication of Acta Eruditorum Ⓣ contained Leibniz's solution to the brachistochrone problem on page 205, Johann Bernoulli's solution on pages 206 to 211, Jacob Bernoulli's solution on pages 211 to 214, and a Latin translation of Newton's solution on page 223. The solution by de L'Hôpital was not published until 1988 when, nearly 300 years later, Jeanne Peiffer presented it as Appendix 1 in [1]. Johann Bernoulli gave the solvers, saying:-
... my elder brother made up the fourth of these, that the three great nations, Germany, England, France, each one of their own to unite with myself in such a beautiful search, all finding the same truth.Johann Bernoulli's solution divides the plane into strips and he assumes that the particle follows a straight line in each strip. The path is then piecewise linear. The problem is to determine the angle of the straight line segment in each strip and to do this he appeals to Fermat's principle , namely that light always follows the shortest possible time of travel. If is the velocity in one strip at angle to the vertical and in the velocity in the next strip at angle to the vertical then, according to the usual sine law
.
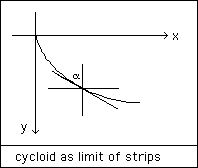
In the limit, as the strips become infinitely thin, the line segments tend to a curve where at each point the angle the line segment made with the vertical becomes the angle the tangent to the curve makes with the vertical. If is the velocity at and is the angle the tangent makes with the vertical then the curve satisfies
= constant.
Galileo had shown that the velocity satisfies
(where is the acceleration due to gravity) and substituting for gives the equation of the curve as
= constant or
Use and to get
for a constant .
The cycloid satisfies this equation. To see this note that
=
so
Johann Bernoulli ended his solution of the brachistochrone problem with these words:-
Before I end I must voice once more the admiration I feel for the unexpected identity of Huygens' tautochrone and my brachistochrone. I consider it especially remarkable that this coincidence can take place only under the hypothesis of Galileo, so that we even obtain from this a proof of its correctness. Nature always tends to act in the simplest way, and so it here lets one curve serve two different functions, while under any other hypothesis we should need two curves ...Despite the friendly words with which Johann Bernoulli described his brother Jacob Bernoulli's solution to the brachistochrone problem (see above), a serious argument erupted between the brothers after the May 1697 publication of Acta Eruditorum Ⓣ. It was Jacob Bernoulli who now challenged his brother. Returning to Galileo's original question regarding the time to reach a vertical line rather than a point he asked:-
Given a starting point and a vertical line, of all the cycloids from the starting point with the same horizontal base, which will allow the point subjected only to uniform gravity, to reach the vertical line most quickly.Johann Bernoulli solved this problem showing that the cycloid which allows the particle to reach the given vertical line most quickly is the one which cuts that vertical line at right angles. There is a wealth of information in the correspondence with Varignon given in [1]. Jacob Bernoulli posed isoperimetric problems to Johann Bernoulli and a bitter dispute arose between the two brothers on these problems which Varignon also became involved in. It was an unpleasant incident, but one of great value to mathematics for the problems being argued about led directly to the founding of the calculus of variations. The quarrel between the Bernoulli brothers is examined in detail in [10] where as well as the mathematical details the author studies the psychological side. He argues convincingly that the bad feeling between them must a started at home with a strict and unfriendly father.
The methods which the brothers developed to solve the challenge problems they were tossing at each other were put in a general setting by Euler in Methodus inveniendi lineas curvas maximi minimive proprietate gaudentes sive solutio problematis isoperimetrici latissimo sensu accepti Ⓣ published in 1744. In this work Euler generalises the problems studies by the Bernoulli brothers but retains the geometrical approach developed by Johann Bernoulli to solve them. He found what has now come to be known as the Euler-Lagrange differential equation for a function of the maximising or minimising function and its derivative.
The idea is to find a function which maximises or minimises a certain quantity where the function is constrained to satisfy certain constraints. For example Johann Bernoulli had posed certain geodesic problems to Euler which, like the brachistochrone problem, were of this type. Here the problem was to find curves of minimum length where the curves were constrained to lie on a given surface. Euler, however, commented that his geometrical approach to these problems was not ideal and it only gave necessary conditions that a solution has to satisfy. The question of the existence of a solution was not solved by Euler's contribution.
Lagrange, in 1760, published Essay on a new method of determining the maxima and minima of indefinite integral formulas. It gave an analytic method to attach calculus of variations type problems. In the introduction to the paper Lagrange gives the historical development of the ideas which we have described above but it seems appropriate to end this article by giving what is in effect a summary of the developments in Lagrange's words:-
The first problem of this type [calculus of variations] which mathematicians solved was that of the brachistochrone, or the curve of fastest descent, which Johann Bernoulli proposed towards the end of the last century. The solution was found by considering special cases, and it was only some time later, in research isoperimetric curves, that the great mathematician of whom we speak and his famous brother Jacob Bernoulli gave some general rules for solving several other problems of the same type. Since, however, the rules were not sufficiently general, the famous Euler undertook the task of reducing all such investigations to a general method which he gave in the work "Essay on a new method of determining the maxima and minima of indefinite integral formulas"; an original work in which the profound science of the calculus shines through. Even so, while the method is ingenious and rich, one must admit that it is not as simple as one might hope in a work of pure analysis ....Lagrange then goes on to describe his introduction of the differential symbol δ. He gives:-
... a method which only requires a straightforward use of the principles if the differential and integral calculus; but I must strongly emphasise that since my method requires that a quantity be allowed to vary in two different ways, so as not to confuse these different variations, I have introduced a new symbol δ into my calculations. In this way δz expresses a difference of z which is different from dz, but which, however, will satisfy the same rules; such that where we have for any equation dz = m dx, we can equally have δz = mδx, and likewise in other cases.
References (show)
- P Costabel and J Peiffer (eds.), Bernoulli, Johann I Der Briefwechsel von Johann I Bernoulli. Band 2 : Der Briefwechsel mit Pierre Varignon : Erster Teil : 1692-1702, Die Gesammelten Werke der Mathematiker und Physiker der Familie Bernoulli (Basel, 1988).
- M de Icaza Herrera, Galileo, Bernoulli, Leibniz and Newton around the brachistochrone problem, Rev. Mexicana Fis. 40 (3) (1994), 459-475.
- P Dietz, Die Ursprünge der Variationsrechnung bei Jakob Bernoulli, Verh. Naturf. Ges. Basel 70 (1959), 81-146.
- H Erlichson, Johann Bernoulli's brachistochrone solution using Fermat's principle of least time, European J. Phys. 20 (5) (1999), 299-304.
- T Koetsier, The story of the creation of the calculus of variations : the contributions of Jakob Bernoulli, Johann Bernoulli and Leonhard Euler (Dutch), in 1985 holiday course : calculus of variations (Amsterdam, 1985), 1-25.
- K Pedersen and K M Pedersen, The early history of the calculus of variations (Danish), Nordisk Mat. Tidskr. 19 (1971), 61-74; 108.
- J Peiffer, Le problème de la brachystochrone à travers les relations de Jean I Bernoulli avec L'Hôpital et Varignon, in Der Ausbau des Calculus durch Leibniz und die Brüder Bernoulli, Basel, 1987, Studia Leibnitiana Sonderheft 17 (Wiesbaden, 1989).
- B Singh and R Kumar, Brachistochrone problem in nonuniform gravity, Indian J. Pure Appl. Math. 19 (6) (1988), 575-585.
- G J Tee, Isochrones and brachistochrones, Neural Parallel Sci. Comput. 7 (3) (1999), 311-341.
- R Thiele, Das Zerwürfnis Johann Bernoullis mit seinem Bruder Jakob, Natur, Mathematik und Geschichte, Acta Hist. Leopold. No. 27 (1997), 257-276.
- G Wanner, Les équations différentielles ont 350 ans, Enseign. Math. (2) 34 (3-4) (1988), 365-385.
- F Williamson, Jr., An historical note on the finite element method, Internat. J. Numer. Methods Engrg. 15 (6) (1980), 930-934.
Additional Resources (show)
Other pages about Brachistochrone problem:
Written by J J O'Connor and E F Robertson
Last Update February 2002
Last Update February 2002