Thomas Harriot
Quick Info
Oxford, England
London, England
Biography
Thomas Harriot was a mathematician and astronomer who founded the English school of algebra. He is described in [10] by Fauvel and Goulding as:-... the greatest mathematician that Oxford has produced ...yet his name has only recently become widely known, and even now his achievements are not fully appreciated by most mathematicians.
We know very little of Harriot's youth. In fact all that is known is that on Friday 20 December 1577 he matriculated at the University of Oxford with an entry in the official records giving his age as seventeen, his father as a plebeian, and his birthplace Oxfordshire. It is from this record that his date of birth is deduced to be 1560 and we know that his father was a "commoner" but the very fact that Harriot was entering Oxford means that it is unlikely that he came from the poorest classes. Despite extensive searches of the Oxfordshire records, no further information concerning his birth or parentage has been found (although a number of possible relatives have been identified).
As an undergraduate at Oxford, Harriot was a student at St Mary's Hall. He became friends with Richard Hakluyt and Thomas Allen, both lecturers at the university, but not at St Mary's Hall. Harriot graduated in 1580 and went to London. It is not clear exactly what he did in his first few years there but, probably from late 1583, he entered Sir Walter Raleigh's service. Hakluyt, dedicating a preface to Raleigh in February 1587, wrote (see for example [4]):-
Ever since you perceived that skill in the navigator's art, the chief ornament of an island kingdom, might attain its splendour amongst us if the aid of the mathematical sciences were enlisted, you have maintained in your household Thomas Harriot, a man pre-eminent in those studies, at a most liberal salary, in order that by his aid you might acquire those noble sciences in your leisure hours ...Harriot wrote a text called Arcticon which was never published and unfortunately no copies have ever been found. This work was essentially his lecture course given at Durham House, Raleigh's lodgings in The Strand in London, where Harriot lived at this time. The lectures were given to the seamen who were being gathered by Raleigh to participate in his expeditions to the New World. Pepper describes the advances in navigational techniques made by Harriot by the time he wrote Arcticon [18]:-
... he solved the problem of reconciling the sun and pole star observations for determining latitude, introduced the idea of using solar amplitude to determine magnetic variation and, as well as improving methods and devices for observation of solar or stellar altitudes, he recalculated tables for the sun's declination on the basis of his own astronomical observations. ... he produced a practical numerical solution of the Mercator problem, most probably by the addition of secants ...As Roche notes in [24]:-
... when combined with new instruments and observational practices it is clear that Raleigh had the best navigational expertise in Europe.It was not only as a navigational instructor that Raleigh employed Harriot. He was involved with the design of the ships for Raleigh's expeditions as well as being involved in the construction of the vessels and selecting the seamen. He was Raleigh's accountant, being responsible for obtaining funding for the expeditions and keeping all the accounts.
Raleigh had the captains Philip Amadas and Arthur Barlowe make an expedition to Roanoke Island off the coast of North Carolina in 1584. Although there is no direct evidence that Harriot made this voyage, Quinn in [23] argues convincingly that he was one of those making this preliminary survey. Harriot was certainly on a voyage to Virginia organised by Raleigh in 1585-86. He sailed from Plymouth on 9 April 1585 on board the Tiger and his observations of a solar eclipse on 19 April have allowed modern scientists to compute the exact position of the ship on that day. Harriot made many notes during his time in the New World, being particularly interested in the language and customs (particularly the eating habits) of the inhabitants. The object of the voyage was to colonise the New World but it was not successful in this aim.
Drake was engaged in sea battles with the Spanish when he learnt that they intended to prevent the British colonists becoming established. Although Drake met up with the colonists, in June 1586 there were severe storms and there was a hurried return to England by Harriot and most of the party. Harriot, together with Drake's ships, landed at Portsmouth in July 1586 and he went immediately to Raleigh to report on the expedition. He published A Briefe and True Report of the New Found Land of Virginia in 1588, a book in which he recommends the smoking of tobacco which he himself had learnt to do in Virginia. However, he also wrote a full account of the voyage which, for some reason, he never published and, despite strenuous attempts to find a copy, seems lost.
By the time Harriot had returned, Raleigh had turned his attention to Ireland. Harriot carried out surveys of the Lismore estate, which was owned by Raleigh, beginning in 1589. Nine years later he was still involved in working out the acreage of plots being leased on the estate. However the political situation was about to change and this would have dramatic implications for Harriot.
Already in the 1590s there were allegations against Raleigh of atheism. The charges were against Raleigh's school and "the conjurer that is master thereof". Harriot felt that this was a reference to him and he discussed the allegations with John Dee (who also felt that the charges might relate to him). There is no reason to believe that Harriot (or Raleigh) were atheists but certainly they were free thinkers and Harriot's scientific approach to the world was, to say the least, viewed with great suspicion by the church. As well as problems caused by allegations, Dee and Harriot discussed scientific and mathematical matters in the 1590s.
Harriot had now moved from working for Raleigh to working for Henry Percy, Duke of Northumberland. The Duke had around him a circle of friends who were scholars, many of whom held a atomistic views. Raleigh's life became so chaotic that Harriot had sought the support of a patron who could provide more stability for his scientific pursuits. In 1595 the Duke made property in Durham over to Harriot and he moved up the social ladder becoming a member of the "landed gentry". Harriot also later held estates in Cornwall and Norfolk. Not long after the Durham transaction, the Duke gave Harriot the use of one of the houses on the estate at Syon (near Kew outside London) which Harriot used both as a residence and as a scientific laboratory.
We certainly know from manuscripts which survive that Harriot was engaged in deep studies of optics at Syon by 1597. Although in [4] it states that he had discovered the sine law of refraction of light before 1597, in fact we now know that the precise date of Harriot's important discovery was July 1601. As with all his other mathematical discoveries, however, Harriot did not publish his findings. It is somewhat ironical, however, that Snell (to whom the discovery of this law is now attributed) was not the first to publish the result. Snell's discovery was in 1621, about 20 years after Harriot's discovery, but the result was not published until Descartes put it in print in 1637.
One of the optical problems which Harriot did study in the 1590s was Alhazen's problem. He gave a solution to Alhazen's problem which involved considering an equivalent problem, namely the problem of the maximum intercept formed between a circle and a diameter of a chord rotating about a point on a circle. The author of [8] conjectures that Harriot may have used infinitesimal techniques in demonstrating the equivalence of these problems, and certainly we know that Harriot introduced ideas later rediscovered by Barrow.
Optics was not the only topic to occupy Harriot during this period. He had been asked by Raleigh in the early 1590s to apply his mathematical skills to the science of gunnery. At this time ideas of the trajectory taken by a projectile were still dominated by Aristotle's thinking. Harriot resolved the forces acting on the projectile into horizontal and vertical components. He understood that air resistance acted throughout the whole flight, and that gravity acted on the vertical component. He came very close to a vector analysis solution of the problem of finding the velocity of the projectile and, certainly by 1607, he came to the conclusion that the path of the projectile was a tilted parabola. He made one error, however, [4]:-
Somehow, he could not force himself to abandon the Aristotelian idea that heavier bodies fell at a faster rate than lighter ones.Other topics which Harriot began to work on before 1600 were problems of chemistry. He worked intensively on chemistry for almost exactly a year from May 1599 to May 1600 and, although his experiments were conducted with a new scientific precision, he made no discoveries of particular note.
Raleigh had been a particular favourite of Elizabeth I and, when she died on 24 March 1603, it was clear that Raleigh's fortunes would change. Perhaps it is less clear that Harriot, by this time not so closely associated with Raleigh, would find problems too. James I became king and he quickly saw Raleigh as someone opposed to his claims to the throne. Henry Percy, the Duke of Northumberland, had taken care to put himself on a good footing with James with a letter of support for him only days before Elizabeth died. In July plots were discovered against James and Raleigh was arrested and charged with high treason.
Raleigh attempted suicide but failed. He then sought Harriot's help in obtaining evidence on his behalf. Raleigh was convicted and sentenced to death by hanging. Poor Harriot was singled out in the judgement as being an atheist and an evil influence. His attempts to help Raleigh had been based on Christian principles (to which undoubtedly he adhered) but this had rather damaged Raleigh as Harriot was seen an atheist using Christian principles for convenience. Harriot was devastated and for about a year undertook no new scientific work as he tried to come to terms with what was happening. Raleigh received a last-minute reprieve from the death sentence but was imprisoned in the Tower of London.
Another plot was to lead to further trouble. On 4 November 1605 Guy Fawkes and others were arrested for attempting to blow up the Houses of Parliament. Four others, including Thomas Percy, the grandson of Henry Percy, were also arrested as the main conspirators. Harriot was held on suspicion of being involved and imprisoned in the Gatehouse. He was interrogated on the charge that he had cast a horoscope of King James in an attempt to use magical powers to influence the King's future. On 27 November Henry Percy, Harriot's patron, was also put in the Tower where he remained until 1621 when he was released. No evidence seems to have been found against Harriot and, although he remained in the Gatehouse for some while writing several letters requesting his release, he was a free man probably by the end of 1605.
As soon as he was released, Harriot returned to his work on optics. He now considered more complex systems and employed Christopher Tooke as a lens grinder from early 1605. His work on light now moved to the dispersion of light into colours. He began to develop a theory for the rainbow and, by 1606, Kepler had heard of the remarkable results on optics achieved by Harriot. Kepler wrote to Harriot, but the correspondence never really achieved any significant exchange of ideas. Perhaps Harriot was too wary of the difficulties that his work had nearly brought on him, or perhaps he did (as he claimed to Kepler) still intend to publish his results if his health permitted.
The appearance of a comet attracted Harriot's attention and turned his scientific mind towards astronomy. He observed a comet on 17 September 1607 from Ilfracombe which would later be identified as Halley's Comet. Kepler had discovered the comet six days earlier but it would be the observations of Harriot and his friend (and student) William Lower which eventually were used by Bessel to compute its orbit.
His astronomy brought back to the fore, Harriot went on to make the earliest telescopic observations in England. On 26 July 1609 at 9 p.m. he sketched the Moon which was at that time 5 days old, viewing it through a telescope with a magnification of 6. He sketched the Moon again a year later on 17 July 1610, by this time he had a telescope giving him a magnification of 10. Soon he had constructed a telescope with a magnification of 20, then by April 1611 he had a 32 magnification telescope.
Harriot observed the moons of Jupiter, although since his first sighting states:-
My first observation of the new planets. I saw but one and that alonehe must have already been aware of Galileo's discovery. As with all his scientific discoveries, Harriot did not publish his results. These observations of Jupiter's moons were made between 17 October 1610 and 26 February 1612.
He was the first to discover sunspots, making 199 observations between 8 December 1610 and 18 January 1613. The first observation of sunspots was made while he was observing Jupiter's moons. From the data he collected he was able to deduce the period of the Sun's rotation. However, around this time his scientific work basically came to an end. He seems to have had his spirits brought low by the deaths of his friends and lost the spirit to continue research (which had brought him much trouble despite his lack of publications).
Of the few pieces of work done by Harriot after 1614, one was his observation of another comet in 1618 (there were three visible comets that year and Harriot observed the third) from Syon House. In 1618 Raleigh, who had been shown the clemency of imprisonment in 1603 rather than death, was put to death. Raleigh was executed on 29 October 1618 in a public execution, with Harriot present to witness the event. However, by this time Harriot was already suffering from the cancer of the nose which eventually led to his death.
The cancer seems to have started around 1613, about the time when Harriot lost interest in pushing forward his mathematical and scientific research. He consulted the top specialist in 1615 who wrote report on the consultation. He described Harriot as (see for example [4]):-
... a man somewhat melancholy. ... A cancerous ulcer in the left nostril eats up the septum of his nose and in proportion to its size holds the lips hard and turned upwards. It has gradually crept well into the nose. This evil the patient has suffered the last two years.Harriot would suffer this "evil" for a further three years before the cancer took his life.
There are a few other major mathematical achievements due to Harriot which we should mention. He exhibited the logarithmic spiral as the stereographic projection of a loxodrome on a sphere, a projection he proved to be conformal. The loxodromes are the straight lines on the Mercator map, which Harriot computed with great precision. In fact in order to achieve this degree of precision, Harriot introduced finite-difference interpolation.
There is an interesting history to a problem which has only recently been solved, yet originated with Harriot. Raleigh asked Harriot to solve certain problems regarding the stacking of cannonballs. On a manuscript dated 12 December 1591 (Sunday), Harriot set out a table to answer Raleigh's questions. He shows how, if the number of cannonballs is given, one can compute the number of cannonballs to be placed in the base of a pyramid with a triangular, square or oblong base. Raleigh posed a second question, which Harriot also answered, namely given the pyramid of cannonballs, compute the number in the pile.
Harriot was too much the mathematician to stop there, however. From a study of how the cannonballs could fill space, he considered the implications for the atomic theory of matter which he believed in. Later, in his correspondence with Kepler about atomic theory, Harriot mentioned the packing problem. Kepler could not solve the problem but he believed that the densest packing of spheres would be attained if in each layer the centres of the spheres were above the centres of the holes in the layer below. This seems intuitively obvious, but resisted proof until 1998 when Thomas Hales of the University of Michigan (with the help of hours of computer generated data) finally proved the conjecture.
The one part of Harriot's work which we have not yet described is the mathematical work for which, in some ways, he is best known, namely his work on algebra. He introduced a simplified notation for algebra and his fundamental research on the theory of equations was far ahead of its time. As an example of his abilities to solve equations, even when the roots are negative or imaginary, we reproduce his solution of an equation of degree 4. The example in question is in his own handwriting and reproduced in [3].
aaaa - 6aa + 136a = 1155
------------------------------------
aaaa -2aa + 1 = 4aa -136a + 1156
aa - 1 = 2a - 34
33 = 2a - aa
aa - 2a = -33
aa - 2a + 1 = +1 - 33
a - 1 = √-32
1 - a = √-32
a = 1 + √-32
a = 1 - √-32
------------------------------------
aa - 1 = 34 - 2a
aa + 2a = 35
aa + 2a + 1 = 1 + 35
a + 1 = √36
a = √36 - 1 = 5
------------------------------------
-a - 1 = √36
a = -√36 - 1 = -7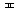 .
.
Harriot invented certain symbols which are used today. However, the symbols < for "less than" and > for "greater than" were not due to Harriot (as is often claimed), but were introduced by the editor of Artis Analyticae Praxis ad Aequationes Algebraicas Resolvendas Ⓣ - Harriot himself used different symbols. There is still scholarly debate on how much Harriot was influenced by Viète, or whether notation and ideas introduced by Viète were learnt by him from Harriot.
As we have seen from the example above, Harriot did outstanding work on the solution of equations, recognising negative roots and complex roots in a way that makes his solutions look like a present day solution. He made the observation that if are the roots of a cubic then the cubic is . This is a major step forward in understanding which Harriot then carried forward to equations of higher degree.
Although he was far ahead of his time, his work had far less influence than it should have done since, as we have remarked repeatedly above, he published no mathematical work in his lifetime. Even his work on algebra Artis Analyticae Praxis ad Aequationes Algebraicas Resolvendas Ⓣ (1631) was published 10 years after his death and was edited by people who did not fully appreciate the depth of his work. For example, it does not discuss negative solutions.
References (show)
- J A Lohne, Biography in Dictionary of Scientific Biography (New York 1970-1990).
See THIS LINK. - Biography in Encyclopaedia Britannica.
http://www.britannica.com/biography/Thomas-Harriot - J N Crossley, The emergence of number (Singapore, 1980).
- J W Shirley, Thomas Harriot : a biography (Oxford, 1983).
- J W Shirley (ed.), A Source book for the study of Thomas Harriot (New York, 1981).
- J W Shirley (ed.), Thomas Harriot : renaissance scientist (Oxford, 1974).
- T F Bloom, Borrowed perceptions : Harriot's maps of the Moon, Journal for the history of astronomy 9 (1978), 117-122.
- P C Fenton, An extremal problem in Harriot's mathematics, Historia Math. 16 (2) (1989), 154-163.
- P C Fenton, Events in the life of the mathematician Thomas Harriot (1560-1621), Austral. Math. Soc. Gaz. 12 (4) (1985), 85-93.
- Thomas Harriot, in J Fauvel, R Flodd and R Wilson (eds.), Oxford figures : 800 years of the mathematical sciences (Oxford, 2000), 56-59.
- J Jacquot, Harriot, Hill, Warner and the new philosophy, in Thomas Harriot : Renaissance scientist (Oxford, 1974), 107-128.
- M Kalmar, Thomas Hariot's 'De reflexione corporum rotundorum' : an early solution to the problem of impact, Arch. History Exact Sci. 16 (3) (1976/77), 201-230.
- J A Lohne, Dokumente zur Revalidierung von Thomas Harriot als Algebraiker, Arch. History Exact Sci. 3 (1966), 185-205.
- J A Lohne, Essays on Thomas Harriot. I. Billiard balls and laws of collision. II. Ballistic parabolas. III. A survey of Harriot's scientific writings, Arch. Hist. Exact Sci. 20 (3-4) (1979), 189-312.
- J A Lohne, Thomas Harriot als Mathematiker, Centaurus 11 (1) (1965/66), 19-45.
- J A Lohne, T Harriot, Centaurus 6 (1959), 113-121.
- J North, Thomas Harriot and the first telescopic observations of sunspots, in Thomas Harriot: Renaissance scientist (Oxford, 1974), 129-165.
- J V Pepper, Harriot's earlier work on mathematical navigation : theory and practice. With an appendix, 'The early development of the Mercator chart', in Thomas Harriot : Renaissance scientist (Oxford, 1974), 54-90.
- J V Pepper, Harriot's manuscript on the theory of impacts, Ann. Of Sci. 33 (2) (1976), 131-151.
- J V Pepper, Harriot's work on the true sea-chart, 1971 Actes XIIe Congrès Internat. d'Histoire des Sciences IV (Histoire des Mathématiques et de la Mécanique) (Paris, 1968),135-138.
- J V Pepper, Some clarifications of Harriot's solution of Mercator's problem, Hist. of Sci. 14 (4) (1976), 235-244.
- J V Pepper, The study of Thomas Harriot's manuscripts. II. Harriot's unpublished papers, History of science 6 (Cambridge, 1967), 17-40.
- D B Quinn, Thomas Harriot and the new world, in Thomas Harriot: Renaissance scientist (Oxford, 1974), 36-53.
- J J Roche, Harriot's 'Regiment of the Sun' and its background in sixteenth-century navigation, British J. Hist. Sci. 14 (48) (1981), 245-261.
- J J Roche, Harriot, Galileo, and Jupiter's satellites, Arch. Internat. Hist. Sci. 32 (108) (1982), 9-51.
- E Rosen, Harriot's science: the intellectual background, in Thomas Harriot: Renaissance scientist (Oxford, 1974), 1-15.
- C J Scriba, Wallis und Harriot, Centaurus 10 (1965), 248-257.
- M Seltman and E Mizzi, Thomas Harriot: father of English algebra?, Math. Intelligencer 19 (1) (1997), 46-49.
- J W Shirley, Sir Walter Ralegh and Thomas Harriot, in Thomas Harriot: Renaissance scientist (Oxford, 1974), 16-35.
- B J Sokol, Thomas Harriot - Sir Walter Raleigh's tutor - on population, Ann. of Sci. 31 (1974), 205-212.
- R C H Tanner, Henry Stevens and the associates of Thomas Harriot, in Thomas Harriot: Renaissance scientist (Oxford, 1974), 91-106.
- R C H Tanner, Nathaniel Torporley's 'Congestor analyticus' and Thomas Harriot's 'De triangulis laterum rationalium', Ann. of Sci. 34 (4) (1977), 393-428.
- R C H Tanner, The ordered regiment of the minus sign : off-beat mathematics in Harriot's manuscripts, Ann. of Sci. 37 (2) (1980), 127-158.
- R C H Tanner, The study of Thomas Harriot's manuscripts. I. Harriot's will, History of science 6 (Cambridge, 1967), 1-16.
- R C H Tanner, Thomas Harriot as mathematician. A legacy of hearsay. I, Physis-Riv. Internaz. Storia Sci. 9 (1967), 235-247.
- R C H Tanner, Thomas Harriot as mathematician. A legacy of hearsay. II, Physis-Riv. Internaz. Storia Sci. 9 (1967), 257-292.
- D T Whiteside, In search of Thomas Harriot, History of Science 13 (1975), 61-62.
Additional Resources (show)
Other pages about Thomas Harriot:
Honours (show)
Honours awarded to Thomas Harriot
Cross-references (show)
- History Topics: An overview of the history of mathematics
- History Topics: Kepler's Planetary Laws
- History Topics: Light through the ages: Ancient Greece to Maxwell
- History Topics: Quadratic, cubic and quartic equations
- History Topics: The fundamental theorem of algebra
- History Topics: Thomas Harriot's manuscripts
- Other: 11th October
- Other: 20th December
- Other: 26th July
- Other: 8th December
- Other: Earliest Uses of Symbols for Variables
- Other: Earliest Uses of Symbols of Operation
- Other: Earliest Uses of Symbols of Relation
- Other: Jeff Miller's postage stamps
- Other: Linda Hall Library links
- Other: London individuals D-G
- Other: London individuals H-M
- Other: Oxford Institutions and Colleges
- Other: Oxford individuals
Written by J J O'Connor and E F Robertson
Last Update January 2000
Last Update January 2000
