A mathematical walk around Bologna

In the summer of 2024 I [EFR] spent a week in Bologna. While there I visited several places with mathematical connections. These visits occurred on different days but I will write this article as if I made the visits during a single walk round the city.

Before starting our walk, however, let us list the mathematicians in the MacTutor Archive who were born in Bologna:

in the 15th Century; Scipione del Ferro, 1465:
in the 16th Century; Lodovico Ferrari, 1522, Rafael Bombelli, 1526, Pietro Cataldi, 1548, Giuseppe Biancani, 1566:
in the 17th Century; Francesco Grimaldi, 1618, Pietro Mengoli, 1626, Eustachio Manfredi, 1674, Gabriele Manfredi, 1681:
in the 18th Century; Laura Bassi, 1711:
in the 19th Century; Umberto Puppini, 1884, Ettore Bortolotti, 1866:
in the 20th Century; Lamberto Cesari, 1910.

Our walk starts on the Via dell'Indipendenza going towards the Piazza del Nettuno.

We cross the Via Rizzoli into the Piazza del Nettuno, pass by the Fontana del Nettuno, a 16th-century fountain with mermaids surrounding the bronze figure of Neptune, and reach the Piazza Maggiore. On our right is the Palazzo d'Accursio, the first place of mathematical interest.

The Palazzo d'Accursio.

The Palazzo d'Accursio or Palazzo Pubblico has been the seat of Bologna's Municipal Authority since 1336. It is named after the Professor of Law, Francesco Accursio, who lived in part of the building in the mid 1200s.

Laura Bassi was taught mathematics by Gaetano Tacconi who was very impressed with the abilities of his pupil and through him she began to gain a reputation among the circle of scholars in Bologna. Several of the scholars who were members of the Academy of Science of Bologna were invited to Bassi's home by Tacconi, himself a member of the Academy, and she entered into disputations with them on philosophical topics. All were impressed by her debating skills and also by the ease with which she assimilated knowledge. One of these learned men was Prospero Lambertini. He had been born in Bologna and awarded a doctorate in theology and law by the University of Rome. He was elevated to cardinal in 1728, and in 1731 he returned to Bologna where he was elevated to an archbishop by Pope Clement XII. Lambertini became Bassi's patron and, to show off his protégé, set up a debate between her and four professors from the University of Bologna on 17 April 1732. The debate was held in the grand Palazzo Pubblico in Bologna and Bassi defended forty-nine philosophical theses. Monique Frize writes in her 2013 book Laura Bassi and Science in 18th Century Europe. The extraordinary life and role of Italy's pioneering female professor:-

Eighteenth century Theses were not pieces of original research as they are now, but answers and a discussion on a set of questions the candidate prepared ahead of time. The candidate first produced written responses in Latin to the questions posed by the professors, which were then read by a committee, and finally defended orally. This particular examination was quite out of the ordinary. Students normally defended their Thesis at the university in the presence of the teaching staff, definitely not in the presence of such a large and prestigious public as was the case on this day. The uniqueness of this event is explained by the fact that the candidate was not a young man, as we would expect, but a young woman, 20 year old Laura Bassi. ... Because of her sex, Laura had to perform in a very public manner in order to obtain recognition of her abilities and knowledge.

In front of us in the Piazza Maggiore is the Basilica of San Petronio which began construction in 1390.

The Basilica of San Petronio.

Construction of the Basilica continued slowly over literally hundreds of years and, as is clearly seen, the facade, which began construction in 1538, remains unfinished today. In this Basilica there are many treasures but the mathematical interest is in the meridian line. These lines were constructed to indicate noon each day, but perhaps more importantly, were used to determine the date of the vernal equinox.

Egnatio Danti's meridian line.

The first meridian line was constructed in the Basilica of San Petronio by the Dominican Egnatio Danti in 1575. Danti had already constructed a meridian line in the church of Santa Maria Novella in Florence to make accurate determinations of the vernal equinox so that he could determine the error in the calendar. In 1574, while in Florence, Danti detected the 11 day error in the calendar. Forced to leave Florence, he moved to Bologna in 1575 and was appointed as Professor of Mathematics at the University of Bologna. He constructed a meridian line across the central nave of the Basilica of San Petronio, with a hole in the wall on the left side of the aisle to allow the sun's rays to enter. In 1653, as construction of the Basilica continued, the wall was removed to enlarge the church and the meridian line could no longer be used. Giovanni Domenico Cassini had been appointed as Professor of Mathematics and Astronomy at the University of Bologna in 1650. He proposed constructing a larger and more accurate meridian instrument in the Basilica of San Petronio.

Cassini's meridian line.

Cassini began to undertake research to find the best position for the hole to let the sun's rays enter so that the meridian line could be constructed without being obstructed by the pillars. He decided that if he positioned the hole in the roof of the fourth vault of the left side aisle, then he could construct the line to just touch the first and second pillars and end in the central nave. The Senate of Bologna approved his plan and work began in the summer of 1655. The hole, made in brass inserted in a block of marble, was placed in the roof. From it he dropped a thin copper wire with a weight at the end to determine the exact point on the floor below. On the summer solstice on 22 June 1655 he traced the line of the image of the sun across the floor enabling him to determine true North. He then constructed his line ensuring it was horizontal using water levels. The work was completed on 21 December 1655 when the winter solstice gave the final extent of the line. The hole is 27.07 metres above the floor and the line length is 2.5 times the height of the hole, and so is over 67.5 metres.

Cassini was invited to Paris in 1668 but made a visit to Bologna in 1695 on his way to Rome. Finding the meridian line was no longer entirely horizontal he initiated repairs. A more major restoration was made by Eustachio Zanotti in 1776. Zanotti (1709-1782) had been born in Bologna and had studied mathematics and astronomy under Eustachio Manfredi. Zanotti had been appointed as director of the Bologna Observatory in 1739. He was asked by the Senate of Bologna to repair Cassini's line. He did such an excellent job that the line we can see today in the Basilica of San Petronio is almost exactly as Zanotti repaired it almost 250 years ago. There are two numbering systems on the line. The height of the hole was taken as 100 units and the line, being 2.5 times that length, was marked every two units up to 250 on one side. On the other side of the line, the time of noon in Italian time is indicated with hours in Roman numerals and minutes in Arabic numerals. Italian time was still in use when Zanotti made his restoration. It divided the day into 24 equal hours, starting with total darkness. The sun set, therefore, 30 minutes before the day began.

After our visit to the Basilica of San Petronio, we exit the church and turning right reach the Via dell'Archiginnasio. We walk down that street, with the Basilica on our right, until we reach the Archiginnasio which used to be the buildings of the University of Bologna. The date of founding of the University is uncertain. Although it was officially granted its charter in 1158, it is claimed that students were taught as part of the university from 1088. The Archiginnasio was the main site of the University of Bologna from 1563 to 1803.

People in MacTutor who taught in Bologna.

The following all taught at Bologna, most as professors at the University but a few as assistants at the University or professors at other institutions in Bologna.

Luca Pacioli (born 1445), Scipione del Ferro (born 1465), Girolamo Cardano (born 1501), Lodovico Ferrari (born 1522), Egnatio Danti (born 1536), Pietro Cataldi (born 1548), Giovanni Magini (born 1555), Giovanni Battista Riccioli (born 1598), Bonaventura Cavalieri (born 1598), Francesco Grimaldi (born 1618), Giovanni Cassini (born 1625), Pietro Mengoli (born 1626), Eustachio Manfredi (born 1674), Vincenzo Riccati (born 1707), Paolo Frisi (born 1728), Luigi Cremona (born 1830), Eugenio Beltrami (born 1835), Cesare Arzelà (born 1847), Salvatore Pincherle (born 1853), Giulio Vivanti (born 1859), Domenico Montesano (born 1863), Ettore Bortolotti (born 1866), Federigo Enriques (born 1871), Ugo Amaldi (born 1875), Giuseppe Vitali (born 1875), Beppo Levi (born 1875), Matteo Bottasso (born 1878), Francesco Severi (born 1879), Umberto Puppini (born 1884), Leonida Tonelli (born 1885), Annibale Comessatti (born 1886), Beniamino Segre (born 1903), Gianfranco Cimmino (born 1908), Lamberto Cesari (born 1910).

Although the majority of these people taught at the University of Bologna after it moved away from the Archiginnasio, there are eleven of these mathematicians who will have taught at the Archiginnasio.

The Archiginnasio.

Entering the Archiginnasio through an impressive portico, you see an internal courtyard that incorporates the former church of Santa Maria dei Bulgari. Perhaps the most striking feature when one enters the Archiginnasio is the literally thousands of coats of arms painted on the walls. Students were divided into nations and those who were elected to be the head of their nation could have their coat of arms on the wall. These give the country or city that the student came from and show how international the University of Bologna was. There are also some busts of those who lectured at the university. The building had two floors and ascending the staircase you can enter two fascinating rooms, the Anatomical Theatre and the Sala Stabat Mater. It is the second of these that contains interesting mathematics books.

The Sala Stabat Mater.

The Sala Stabat Mater has a wonderful collection of old science books. You might think the name Stabat Mater Room is a bit strange so I will explain why it has this name. On the evening of 18 March 1842 Gioachino Rossini's Stabat Mater was performed under the direction of Gaetano Donizetti, with repeats on the two following evenings, in what was then called the great hall of the Archiginnasio. It was the first Italian performance and Rossini himself, who lived in Bologna at that time, had wanted it to enable a fund to be set up to subsidise Bolognese musicians who were in poverty. Here are two views of the room in the summer of 2024.

Here is a picture of me standing in front of the Mathematics Section of the Stabat Mater Room.

The collection of mathematics books is fascinating with some major works such as Leonard Euler's Élémens d'algèbra avec des notes et des additions (1774) and Isaac Newton's Philosophiae Naturalis Principia Mathematica Ⓣ (1739-42), but it also contains lesser known works such as Girolamo Saladini's Nuovo metodo delle proporzioni aritmetica, geometrica ed armonica Ⓣ (1761) and Francesco Cardinali's Elementi d'aritmetica Ⓣ (1808).

We give information about ten of the mathematics books in the Stabat Mater Room at THIS LINK.

Sadly the library in the Sala Rusconi, a former lecture hall, is not open to the public but there is a view through a door between the library and the Sala Stabat Mater.

The library contains rare valuable books and manuscripts and it would be wonderful to be able to seek items of interest there. These works were collected by Pietro Giacomo Rusconi (1865-1916) who had no direct heirs. After his widow Luisa Verzaglia died in 1919 she left everything to the Municipality of Bologna. In 1922 the printed material "most valuable either for the antiquity of the editions, or for their value or for the particularities of the various volumes" was placed in a room of the Archiginnasio which was named after the donor Rusconi whose portrait is seen under the name "Sala Rusconi".

Leaving the Archiginnasio, we go back along the Via dell'Archiginnasio to the Piazza Maggiore continuing until we reach the Via Rizzoli. Turning right along the Via Rizzoli we immediately see ahead Le due Torri, the Two Towers, one with a rather severe lean which is the Garisenda tower. This tower is in danger of collapse so the area round the towers is cordoned off. The tallest of Le due Torri, the Asinelli tower built between 1109 and 1119, is 97.2 metres tall. It has mathematical interest which we now relate.

The Asinelli tower.

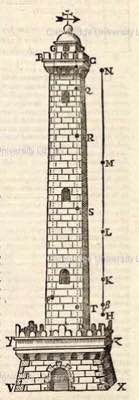
Galileo had published Discorsi e dimostrazioni matematiche intorno a due nuove scienze Ⓣ in 1638. In this work Galileo shows that the distances travelled by naturally accelerating objects are proportional to the squares of the time taken. He also shows that objects accelerate vertically downward at a constant rate, regardless of their mass, due to the effects of gravity. Giovanni Battista Riccioli carried out experiments, beginning in 1640, to verify or disprove Galileo's claims. He was assisted in this work by Francesco Maria Grimaldi who he had taught in Parma. Both had moved to Bologna in 1636 and after reading Galileo's Discorsi Ⓣ they decided to create an accurate timing device needed for the experiments. First, Grimaldi and Riccioli calibrated a pendulum by getting it to swing for 24 hours (measured by the star Arcturus crossing the meridian line). They used this 3 foot pendulum to calibrate a shorter pendulum to use in timing. They then dropped balls of wood and of lead from various heights from the Asinelli tower. A nice addition to the accuracy was obtained by getting a group of musical monks to chant in time with the swinging pendulum to aid with the timing. The experiment did not confirm Galileo's result for, as one might expect, the lead balls reached the ground before the wooden ones in all the experiments using different heights on the tower. He claimed that the reason the experiments had come up with different results was that Galileo, and others making the same claims, had only dropped weights from 50 or 100 feet while his experiments had used the 312 foot high Asinelli tower. Riccioli describes these experiments in Almagestum novum Ⓣ (1651) illustrating it with this drawing of the Asinelli tower. He did claim, however, to have verified Galileo's square law for falling objects.

There is a second reason to be interested in experiments using the Asinelli tower. In 1789 Giovanni Battista Guglielmini (1763-1817) published Riflessioni sopra un nuovo esperimento in prova del diurno moto della terra Ⓣ describing experiments to prove the rotation of the earth. The experiments were conducted using the Asinelli tower. He then carried out further experiments in 1791-92 which he described in De diuturno terrae motu experimentis physico-mathematicis confirmato Ⓣ (1792). The following description is taken from the Linda Hall Library:

The practical difficulties were daunting. The predicted displacements, even in a tall tower like the Asinelli, were small - less that one inch. The Asinelli tower is over one degree out of vertical, so one would need a plumb bob almost 300 ft long to establish verticality. A ball dropped from a tower might be buffeted about by winds, and even the passage of carriages in the streets could vibrate the building and affect the results. To mitigate the wind, Guglielmini opted to drop his balls inside the tower, which meant he had to cut holes through several floors. To lessen the passing-carriage effect, he worked in the middle of the night. He did experiments for over a year, but only a few of them were useful, because normally something was causing either the air or the tower or the plumb bob to be unstable. He also found out early on that even when conditions were good, the results varied dramatically, leading him to suspect that their method for dropping the balls was faulty. So he invented a device for just that purpose, which would drop a ball without imparting a sideways motion or spin. By 1792, Guglielmini finally had some good results, which showed a small displacement to the east and an even smaller displacement to the south.

Guglielmini's experiments had defects, both in his theory and in his experiments themselves. The experiments did, however, interest Laplace who found mathematical errors in Guglielmini's theory. Surprisingly, however, Guglielmini's book was a factor in the Roman Catholic Church lifting its ban on Copernican theory in 1822.

After the visit to the two towers, go down the Strada Maggiore, passing Rossini's house were he lived for three years, to arrive at the Museo internazionale e biblioteca della musica in the magnificent Palazzo Aldini Sanguinetti.

International Museum and Library of Music.

After ascending the staircase and entering the Music Museum, one is immediately stunned by the beauty of the Palazzo Aldini Sanguinetti in which the Museum is housed.

Reading the information panels on the walls soon shows that there might be items of mathematical interest in this museum.

A number of books written by mathematicians are on display, in particular books by Marin Mersenne are on display in this room of the museum.

I found five books by mathematicians displayed in the Music Museum. These are: René Descartes, Musicae compendium Ⓣ (1683); Pietro Mengoli, Speculationi di musicae Ⓣ (1670); Marin Mersenne, Harmonie universelle Ⓣ (1636); Marin Mersenne, Cogitata physico-mathematica Ⓣ (1644); and Euclid, Rudimenta Musices Ⓣ (1557). For more information about these books, see THIS LINK.

After leaving the Museo internazionale e biblioteca della musica, we continue along the Strada Maggiore until we reach the Basilica of Santa Maria dei Servi which, although I could not find a plaque about this, is associated with one of the most important mathematical discoveries of the 15th century.

The Basilica of Santa Maria dei Servi.

Scipione del Ferro (1465-1526) held the Chair of Arithmetic and Geometry at the University of Bologna. He taught at the university from 1496. At this time the University of Bologna had different departments in different areas of the city and only brought these together in 1565 when the University began to operate from the Archiginnasio. In the 15th century the University of Bologna was called the Studium and a professorship of Mathematics and Astronomy had been established as part of the Università degli Artisti in 1451.

Luca Pacioli lectured at Bologna in 1501-02 and may have discussed solving cubic equations with del Ferro. We know that del Ferro discovered the algebraic solution to the cubic equation in 1505, but did not explain his method. He may even have used this knowledge when he regularly took part in mathematical contests under the portico of the Basilica of Santa Maria dei Servi in Bologna and astonished the public and his colleagues by solving problems that they suggested. These mathematical challenges gave credit and prestige to the winners who, therefore, enjoyed the support of noblemen. Thanks to his success in the Basilica of Santa Maria dei Servi contests, from 1496 to 1510 Scipione del Ferro's salary increased from 25 to 150 lira.

I stood under the portico of the Basilica of Santa Maria dei Servi and wondered what it would be like to take part in such mathematical contests. I don't know if I'd have liked to be in such a contest but certainly I'd have enjoyed watching it. If such contests were held today, would members of the public come to watch? At first I thought, "No chance!", but then I thought about the thousands who watch University Challenge on British television but probably don't know the answer to a single question.

The most famous of these mathematical contests was that between Antonio Maria Fior (a student of del Ferro) and Niccolò Tartaglia in February 1535 concerning solving cubic equations algebraically. Here is the description of this contest as given by the winner Tartaglia:

Zuanne de Tonini da Coi: I have heard that some time ago you entered into a disputation with Master Antonio Maria Fior and that in the end you reached agreement whereby he was to propound thirty problems for you, each of a different kind, set down in writing and sealed, to be deposited with Master Per Iacomo di Zambelli, notary, and similarly you would propound thirty problems for him, each of a different kind also. This you both did, fixing a term of forty or fifty days for each of you to solve these problems and agreeing that whichever of you within that time should be adjudged to have solved the greater number of the questions you had been given would take the honours, together with some small reward you suggested for each problem. And it has been reported to me, and confirmed by Fina a Bressa, that you solved all thirty of his problems in the space of two hours. I find that hard to believe.

Tartaglia: All that you have been told or had reported to you is true. And the reason why I was able to solve his thirty problems in so short a time is that all thirty concerned work involving the algebra of unknowns and cubes equalling numbers. He did this believing that I would be unable to solve any of them, because Brother Luca asserts in his treatise that it is impossible to solve such a problem by any general rule. However, by good fortune, only eight days before the time fixed for collecting the two sets of thirty sealed problems, I had discovered the general rule for such expressions. All the problems I propounded for him were indeed each of a different kind. I did this in order to show him my versatility, and that my grounding lay not merely in one or two, or even three, private discoveries of mine, or in secrets, although I had kept them to myself for greater safety. Moreover, I could have set him another thousand, not just thirty; instead, as agreed, I propounded all thirty each of a different kind, to show that I thought little of him and had no cause whatever to fear him.

Girolamo Cardano heard of Tartaglia's victory in the contest and persuaded him to divulge the method which Cardano published in his masterpiece Ars Magna (1545). At this time Cardano was in Milan but he lived in Bologna between the time his son was executed for murdering his wife in 1560 and the time he himself was arrested by the Inquisition in 1570. In Bologna Cardano taught at the university and must have been one of the first mathematics professors to teach in the Archiginnasio when it opened in 1565.

One other 'mathematical' visit, which does not quite fit with the walk described above, was to the 'Museum of Illusions, between art and science' in the Palazzo Belloni on Via de' Gombruti.

Museum of Illusions.

The Museum of Illusions is great fun and illustrates clearly that we should never quite believe what we 'see'. Much, of course, is based on the fact that we don't actually see anything. Our eyes convert light signals into electrical signals which are sent to the brain. The brain then interprets these signals and we believe we are seeing what is actually in front of us. Here is an example. It is the Necker Cube due to the Swiss crystallographer Louis Albert Necker (1786-1861) in 1832. It is simply the projection of a 3-dimensional cube onto a 2-dimensional surface.

Our brains can interpret the two dimensional illustration of a cube as being 3-dimensional. There are, however, two different 3-dimensional versions depending on which face the brain thinks is in front.

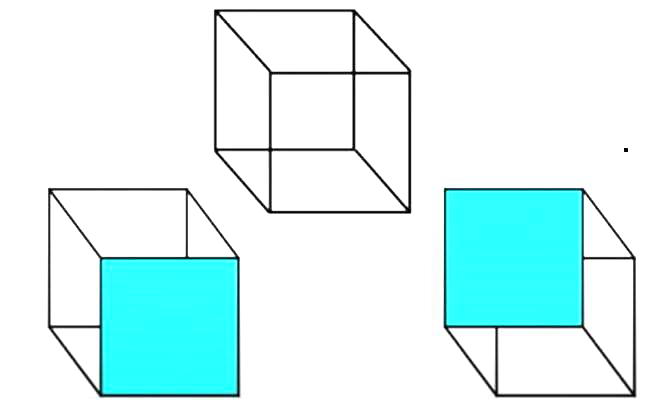
It you stare hard at this picture then you see the front face change. Perhaps it didn't work for you. The following picture shows the two versions.

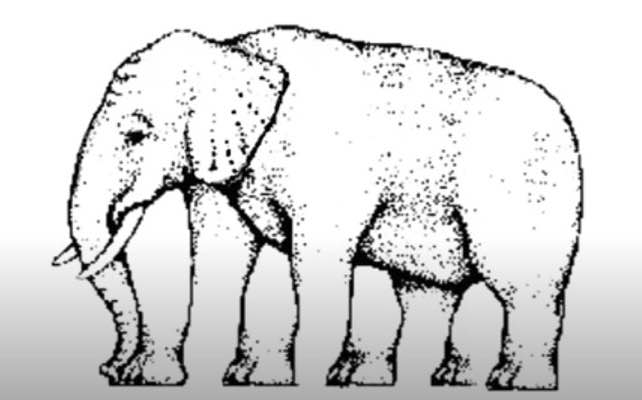
Then there are examples in the Museum like the Impossible Elephant. Again our brain is trying to make a 3-dimensional elephant but gets confused.

Another example from the Museum where the brain will swap from one picture to another is the following. Is it a duck or a hare?

A slightly different type of illusion is the rotating circles where somehow our brain believes the circles rotate.

The only mathematician I saw named in the Museum of Illusions was Augustin Jean Fresnel. There is an exhibit of a Fresnel lens which was originally invented by him for use in lighthouses. The exhibit suggests you take a selfie through the Fresnel lens. I didn't try this so I don't have a picture to show.

Additional Resources (show)

Other pages about Bologna walk:

Written by J J O'Connor and E F Robertson
Last Update September 2024