A history of Topology

Topological ideas are present in almost all areas of today's mathematics. The subject of topology itself consists of several different branches, such as point set topology, algebraic topology and differential topology, which have relatively little in common. We shall trace the rise of topological concepts in a number of different situations.

Perhaps the first work which deserves to be considered as the beginnings of topology is due to Euler. In 1736 Euler published a paper on the solution of the Königsberg bridge problem entitled Solutio problematis ad geometriam situs pertinentis Ⓣ. The title itself indicates that Euler was aware that he was dealing with a different type of geometry where distance was not relevant.

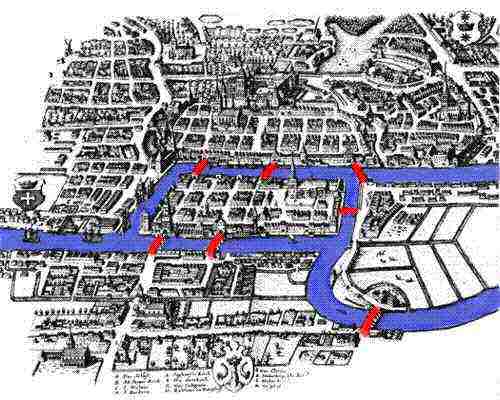
Konigsberg colour

Here is a diagram of the Königsberg bridges

The paper not only shows that the problem of crossing the seven bridges in a single journey is impossible, but generalises the problem to show that, in today's notation,

A graph has a path traversing each edge exactly once if exactly two vertices have odd degree.

The next step in freeing mathematics from being a subject about measurement was also due to Euler. In 1750 he wrote a letter to Christian Goldbach which, as well as commenting on a dispute Goldbach was having with a bookseller, gives Euler's famous formula for a polyhedron

v - e + f = 2

where

v

is the number of vertices of the polyhedron,

e

is the number of edges and

f

is the number of faces. It is interesting to realise that this, really rather simple, formula seems to have been missed by Archimedes and Descartes although both wrote extensively on polyhedra. Again the reason must be that to everyone before Euler, it had been impossible to think of geometrical properties without measurement being involved.

Euler published details of his formula in 1752 in two papers, the first admits that Euler cannot prove the result but the second gives a proof based dissecting solids into tetrahedral slices. Euler overlooks some problems with his remarkably clever proof. In particular he assumed that the solids were convex, that is a straight line joining any two points always lies entirely within the solid.

The route started by Euler with his polyhedral formula was followed by a little known mathematician Antoine-Jean Lhuilier (1750 -1840) who worked for most of his life on problems relating to Euler's formula. In 1813 Lhuilier published an important work. He noticed that Euler's formula was wrong for solids with holes in them. If a solid has

g

holes the Lhuilier showed that

v - e + f = 2 - 2g

This was the first known result on a topological invariant.

Möbius published a description of a Möbius band in 1865. He tried to describe the 'one-sided' property of the Möbius band in terms of non-orientability. He thought of the surface being covered by oriented triangles. He found that the Möbius band could not be filled with compatibly oriented triangles.

Johann Benedict Listing (1802-1882) was the first to use the word topology. Listing's topological ideas were due mainly to Gauss, although Gauss himself chose not to publish any work on topology. Listing wrote a paper in 1847 called Vorstudien zur Topologie Ⓣ although he had already used the word for ten years in correspondence. The 1847 paper is not very important, although he also introduces the idea of a complex, since it is extremely elementary. In 1861 Listing published a much more important paper in which he described the Möbius band (4 years before Möbius) and studied components of surfaces and connectivity.

Listing was not the first to examine connectivity of surfaces. Riemann had studied the concept in 1851 and again in 1857 when he introduced the Riemann surfaces. The problem arose from studying a polynomial equation

f (w, z) = 0

and considering how the roots vary as

w

and

z

vary. Riemann introduced Riemann surfaces, determined by the function

f (w, z)

, so that the function

w(z)

defined by the equation

f (w, z) = 0

is single valued on the surfaces.

Jordan introduced another method for examining the connectivity of a surface. He called a simple closed curve on a surface which does not intersect itself an irreducible circuit if it cannot be continuously transformed into a point. If a general circuit

c

can be transformed into a system of irreducible circuits

a_{1}, a_{2}, ...., a_{n}

so that

c

describes

a_{i} m_{i}

times then he wrote

c = m_{1}a_{1} + m_{2}a_{2} + ....+ m_{n}a_{n}

The circuit

c

is reducible if

m_{1}a_{1} + m_{2}a_{2} + ....+ m_{n}a_{n} = 0

. (*)

A system of irreducible circuits

a_{1}, a_{2}, ...., a_{n}

is called independent if they satisfy no relation of the form (*) and complete if any circuit can be expressed in terms of them. Jordan proved that the number of circuits in a complete independent set is a topological invariant of the surface.

Listing had examined connectivity in three dimensional Euclidean space but Betti extended his ideas to

n

dimensions. This is not as straightforward as it might appear since even in three dimensions it is possible to have a surface that cannot be reduced to a point yet closed curves on the surface can be reduced to a point. Betti's definition of connectivity left something to be desired and criticisms were made by Heegaard.

The idea of connectivity was eventually put on a completely rigorous basis by Poincaré in a series of papers Analysis situs Ⓣ in 1895. Poincaré introduced the concept of homology and gave a more precise definition of the Betti numbers associated with a space than had Betti himself. Euler's convex polyhedra formula had been generalised to not necessarily convex polyhedra by Jonquières in 1890 and now Poincaré put it into a completely general setting of a

p

-dimensional variety

V

.

Also while dealing with connectivity Poincaré introduced the fundamental group of a variety and the concept of homotopy was introduced in the same 1895 papers.

A second way in which topology developed was through the generalisation of the ideas of convergence. This process really began in 1817 when Bolzano removed the association of convergence with a sequence of numbers and associated convergence with any bounded infinite subset of the real numbers.

Cantor in 1872 introduced the concept of the first derived set, or set of limit points, of a set. He also defined closed subsets of the real line as subsets containing their first derived set. Cantor also introduced the idea of an open set another fundamental concept in point set topology.

Weierstrass in 1877 in a course of unpublished lectures gave a rigorous proof of the Bolzano-Weierstrass theorem which states

A bounded infinite subset S of the real numbers possesses at least one point of accumulation p, i.e. p satisfies the property that given any ε > 0 there is an infinite sequence (pn ) of points of S with $| p - p_{n} | < \epsilon$ .

Hence the concept of neighbourhood of a point was introduced.

Hilbert used the concept of a neighbourhood in 1902 when he answered in the affirmative one of his own questions, namely

Is a continuous transformation group differentiable?

In 1906 Fréchet called a space compact if any infinite bounded subset contains a point of accumulation. However Fréchet was able to extend the concept of convergence from Euclidean space by defining metric spaces. He also showed that Cantor's ideas of open and closed subsets extended naturally to metric spaces.

Riesz, in a paper to the International Congress of Mathematics in Rome (1909), disposed of the metric completely and proposed a new axiomatic approach to topology. The definition was based on an set definition of limit points, with no concept of distance. A few years later in 1914 Hausdorff defined neighbourhoods by four axioms so again there were no metric considerations. This work of Riesz and Hausdorff really allows the definition of abstract topological spaces.

There is a third way in which topological concepts entered mathematics, namely via functional analysis. This was a topic which arose from mathematical physics and astronomy, brought about because the methods of classical analysis were somewhat inadequate in tackling certain types of problems. Jacob Bernoulli and Johann Bernoulli invented the calculus of variations where the value of an integral is thought of as a function of the functions being integrated.

Hadamard introduced the word 'functional' in 1903 when he studied linear functionals

F

of the form

F(f) = lim \int f (x) g_{n}(x) dx

where the limit is taken as

n \rightarrow ∞

and the integral is from

a

b

. Fréchet continued the development of functional by defining the derivative of a functional in 1904.

Schmidt in 1907 examined the notion of convergence in sequence spaces, extending methods which Hilbert had used in his work on integral equations to generalise the idea of a Fourier series. Distance was defined via an inner product. Schmidt's work on sequence spaces has analogues in the theory of square summable functions, this work being done also in 1907 by Schmidt himself and independently by Fréchet.

A further step in abstraction was taken by Banach in 1932 when he moved from inner product spaces to normed spaces. Banach took Fréchet's linear functionals and showed that they had a natural setting in normed spaces.

Poincaré developed many of his topological methods while studying ordinary differential equations which arose from a study of certain astronomy problems. His study of autonomous systems

\Large\frac{dx}{dt}\normalsize = f (x, y) , \Large\frac{dy}{dt}\normalsize = g(x, y)

involved looking at the totality of all solutions rather than at particular trajectories as had been the case earlier. The collection of methods developed by Poincaré was built into a complete topological theory by Brouwer in 1912.

References (show)

E Breitenberger, Gauss und Listing : Topologie und Freundschaft, Gauss-Ges. Göttingen Mitt. 30 (1993), 3-56.
D E Cameron, The birth of Soviet topology, Proceedings of the 1982 Topology Conference, Topology Proc. 7 (2) (1982), 329-378.
J W Dauben, Topology : Invariance of dimension, in I Grattan-Guinness (ed.), Companion Encyclopedia of the History and Philosophy of the Mathematical Sciences (London, 1994), 939-949.
J W Dauben, The invariance of dimension : Problems in the early development of set theory and topology, Historia Mathematica 2 (1975), 273-288.
D C Demaria, Francesco Severi's contribution to topology (Italian), Proceedings of the mathematical congress in celebration of the one hundredth birthday of Guido Fubini and Francesco Severi, Atti Accad. Sci. Torino Cl. Sci. Fis. Mat. Natur. 115 (1981), 161-165.
J Dieudonné, A History of Algebraic and Differential Topology, 1900-1960 (Basel, 1989).
J Dieudonné, Une brève histoire de la topologie, in Development of mathematics 1900-1950 (Basel, 1994), 35-155.
J Dieudonné, The beginnings of topology from 1850 to 1914, in Proceedings of the conference on mathematical logic 2 (Siena, 1985), 585-600.
J J Fingerman, The historical and philosophical significance of the emergence of point set topology (PhD Thesis, University of Chicago, 1981).
V L Hansen, From geometry to topology (Danish), Normat 36 (2) (1988), 48-60.
D M Johnson, The problem of the invariance of dimension in the growth of modern topology. I, Archive for History of Exact Sciences 20 (2) (1979), 97-188.
D M Johnson, The problem of the invariance of dimension in the growth of modern topology. II, Archive for History of Exact Sciences 25 (2-3) (1981), 85-267.
S Lefschetz, The early development of algebraic topology, Bol. Soc. Brasil. Mat. 1 (1) (1970), 1-48.
J H Manheim, The genesis of point set topology (New York, 1964).
E Scholtz, Topology : Geometric, algebraic, in I Grattan-Guinness (ed.), Companion Encyclopedia of the History and Philosophy of the Mathematical Sciences (London, 1994), 927-938.
J C Stillwell, Classical Topology and Combinatorial Group Theory (New York, 1980).
A Weil, Riemann, Betti and the birth of topology, Archive for History of Exact Sciences 20 (2) (1979), 91-96.

Additional Resources (show)

Other websites about Topology history:

C Ponce (A Spanish translation of this article)

Written by J J O'Connor and E F Robertson
Last Update May 1996